Sin(x) Maclaurin Series: The Secret You Need to Know!

The fascinating world of calculus offers powerful tools for approximating complex functions, and the sinx maclaurin series stands out as a prime example. This infinite series representation of the sine function, meticulously developed and rigorously proven, finds applications in various fields, from physics simulations to the design of electrical engineering circuits. Understanding the derivation and behavior of the sinx maclaurin series is crucial for anyone seeking a deeper grasp of mathematical modeling.

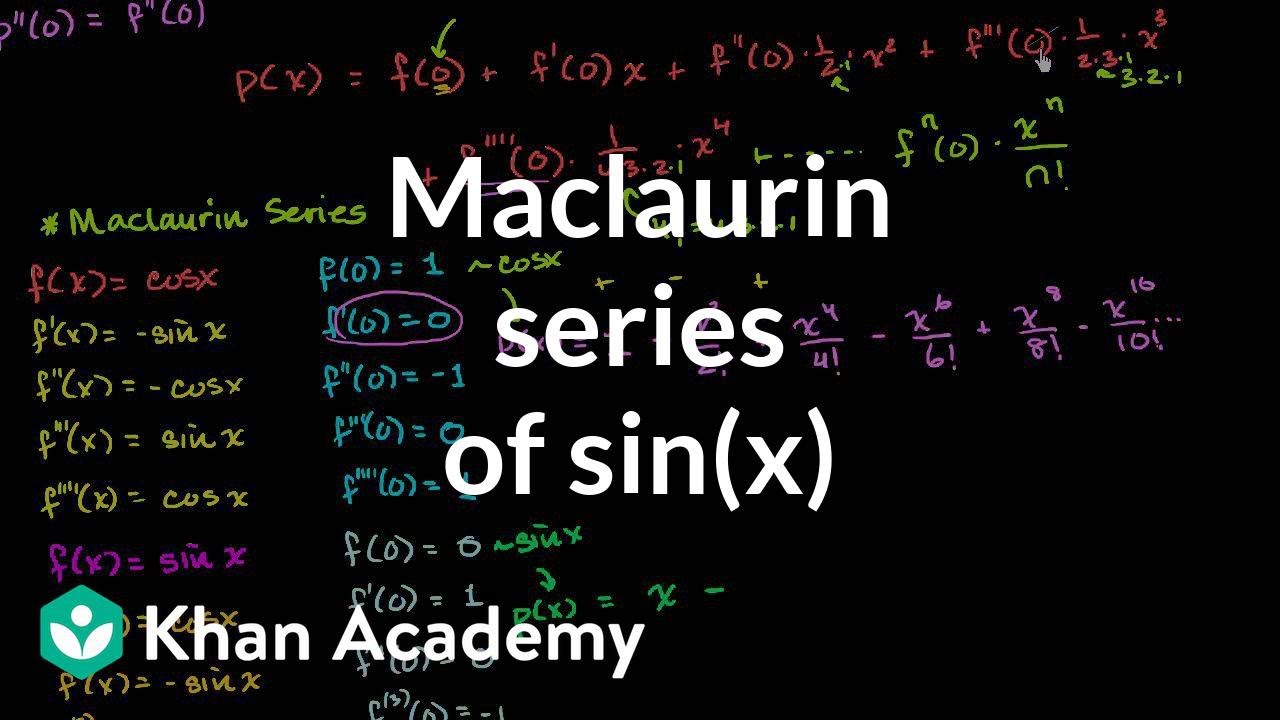
Image taken from the YouTube channel Khan Academy , from the video titled Maclaurin series of sin(x) | Series | AP Calculus BC | Khan Academy .

The sine function, denoted as sin(x), is a cornerstone of mathematics and physics, weaving its way through diverse fields like trigonometry, calculus, wave mechanics, and signal processing. Its periodic nature describes oscillations and cyclical phenomena, making it indispensable for modeling real-world processes. But what happens when we need to understand its behavior in scenarios where direct computation is difficult or impossible? That's where the Maclaurin series comes into play, offering a powerful alternative representation.

This exploration delves into the Maclaurin series representation of sin(x), a journey that unveils the function's intricate structure through the lens of infinite polynomials. We aim to provide a comprehensive explanation of this series, suitable for anyone with a basic understanding of calculus.

Why the Maclaurin Series Matters

The Maclaurin series is not merely an academic curiosity; it's a practical tool with far-reaching implications.

It allows us to approximate the value of sin(x) using a polynomial, which is particularly useful when dealing with very small values of x or when computational resources are limited.

Moreover, the series provides insights into the fundamental nature of the sine function, revealing how it can be constructed from an infinite sum of terms.

Purpose and Preview

The primary goal of this discussion is to elucidate the Maclaurin series expansion of sin(x). We'll carefully unpack the underlying principles and show how this series emerges from the function's derivatives.

While the derivation itself is fascinating, its true power lies in its applications. The series allows us to approximate sine values with increasing accuracy and provides a foundation for more advanced scientific computations.

Teasing the Applications

Consider, for instance, the motion of a pendulum. For small angles, the sine of the angle can be accurately approximated by the angle itself, a simplification directly derived from the Maclaurin series. This approximation simplifies the equations of motion, making them solvable.

Similarly, in signal processing, the Maclaurin series is used to analyze and manipulate complex waveforms. The series allows engineers to decompose signals into their constituent frequencies, enabling techniques like filtering and compression. These are just a few glimpses into the vast potential of this seemingly abstract mathematical concept.

The exploration of the sin(x) Maclaurin series promises to be insightful, but before we dive into the specifics, let’s solidify the foundational concepts upon which this mathematical edifice rests. Understanding the Maclaurin series itself, its relationship to the more general Taylor series, and the underlying principles of calculus is essential for appreciating the power and elegance of this representation. This groundwork will ensure a firm grasp of the derivation and application of the sin(x) Maclaurin series that follows.

Maclaurin Series: A Foundation of Calculus

At the heart of our exploration lies the Maclaurin series, a powerful tool for representing functions as infinite polynomials.

It allows us to approximate the value of complex functions using simpler, more manageable expressions.

Let's unpack the definition and the underlying concepts.

Defining the Maclaurin Series

The Maclaurin series is a special type of Taylor series that provides a polynomial representation of a function f(x) around x = 0.

The general formula for the Maclaurin series is:

Where:

• f(0), f'(0), f''(0), etc., represent the function and its derivatives evaluated at x = 0.
• n! denotes the factorial of n (e.g., 5! = 5 × 4 × 3 × 2 × 1).
• Σ represents the summation from n = 0 to infinity.
• f^(n)(0) means the nth derivative of f evaluated at 0.

Components of the Formula

The Maclaurin series formula might look intimidating at first, but let's break it down:

• Derivatives: Each term in the series involves a derivative of the function f(x) evaluated at zero. The first term uses the function itself f(0), the second term uses the first derivative f'(0), the third term uses the second derivative f''(0), and so on. These derivatives capture the rate of change of the function at that specific point.

• Factorials: The denominator of each term contains a factorial. The factorial ensures that the coefficients of the polynomial terms scale appropriately. This scaling is vital for the series to accurately represent the function.

Maclaurin and Taylor Series: A Closer Look

The Maclaurin series is intimately related to the Taylor series.

In fact, the Maclaurin series is simply a special case of the Taylor series.

The Taylor series represents a function f(x) around an arbitrary point a, while the Maclaurin series specifically centers the representation at a = 0.

The general formula for the Taylor series is:

Notice that when a = 0, the Taylor series reduces to the Maclaurin series.

The Role of Calculus: Derivatives

The Maclaurin series heavily relies on calculus, specifically the concept of derivatives.

Derivatives measure the instantaneous rate of change of a function, and they are essential for constructing the polynomial approximation.

Each term in the Maclaurin series is directly determined by the value of a derivative of the function at x = 0.

The more derivatives we include, the more accurately the polynomial approximates the original function.

Infinite Series: The Building Blocks

The Maclaurin series is an infinite series, meaning it's a sum of infinitely many terms.

While we can't compute an infinite sum directly, we can approximate it by adding a finite number of terms.

The more terms we include, the better the approximation becomes, provided the series converges.

The convergence of the series is a crucial aspect, as it determines whether the series approaches a finite value and accurately represents the function.

Polynomial Approximation: Simplifying Complexity

One of the primary reasons for using the Maclaurin series is its ability to approximate complex functions using polynomials.

Polynomials are simple to evaluate, differentiate, and integrate, making them incredibly useful for various mathematical and computational tasks.

By representing a function as a polynomial, we can simplify calculations and gain insights into the function's behavior.

This is especially valuable when dealing with functions that are difficult to compute directly or that do not have closed-form expressions.

Deriving the concepts that underpin the Maclaurin series offers a solid theoretical grounding, the true magic unfolds when we apply this knowledge to a specific function. Let’s now turn our attention to the sine function, sin(x), and embark on a step-by-step journey to derive its Maclaurin series representation. This process will not only illuminate the power of the Maclaurin series but also provide a tangible example of how calculus can be used to express complex functions in a surprisingly elegant form.

Deriving the Sin(x) Maclaurin Series: Step-by-Step

The derivation of the sin(x) Maclaurin series is a beautiful illustration of how calculus can unlock the hidden polynomial nature of trigonometric functions.

The process involves calculating successive derivatives, identifying repeating patterns, and carefully applying the Maclaurin series formula. Let's unpack each stage of this mathematical journey.

Calculating the Derivatives of sin(x)

The first step in deriving the Maclaurin series for sin(x) is to calculate its derivatives. This is crucial because the Maclaurin series formula relies on the function's derivatives evaluated at x = 0.

We'll begin by finding the first few derivatives of sin(x) and observe the emerging pattern.

• f(x) = sin(x)
• f'(x) = cos(x)
• f''(x) = -sin(x)
• f'''(x) = -cos(x)
• f''''(x) = sin(x)

Notice how the derivatives cycle through sin(x), cos(x), -sin(x), and -cos(x). This cyclical pattern is key to understanding the resulting Maclaurin series.

Identifying the Pattern in the Derivatives

The cyclical nature of the derivatives of sin(x) is fundamental to simplifying the Maclaurin series.

After four differentiations, the pattern repeats itself. This repetition allows us to express the Maclaurin series in a concise and elegant form.

To apply the Maclaurin series formula, we need to evaluate these derivatives at x = 0:

• f(0) = sin(0) = 0
• f'(0) = cos(0) = 1
• f''(0) = -sin(0) = 0
• f'''(0) = -cos(0) = -1
• f''''(0) = sin(0) = 0

The values at x = 0 are either 0 or ±1, further simplifying the terms in the series. The derivatives evaluated at zero give us the coefficients we need for our Maclaurin series.

Applying the Maclaurin Series Formula

Now that we have the derivatives of sin(x) and their values at x = 0, we can apply the Maclaurin series formula:

f(x) = f(0) + f'(0)x + (f''(0)x^2)/2! + (f'''(0)x^3)/3! + (f''''(0)x^4)/4! + ...

Substituting the values we calculated, we get:

sin(x) = 0 + 1x + (0x^2)/2! + (-1x^3)/3! + (0x^4)/4! + (1

**x^5)/5! + ...

Simplifying this, we observe that the terms with even powers of x vanish since their corresponding derivatives are zero at x = 0.

The Resulting Sin(x) Maclaurin Series

After simplification, the Maclaurin series for sin(x) emerges as an infinite sum of odd powers of x:

sin(x) = x - (x^3)/3! + (x^5)/5! - (x^7)/7! + (x^9)/9! - ...

This can be represented more compactly using summation notation:

sin(x) = Σ (-1)^n** (x^(2n+1)) / (2n+1)! where n ranges from 0 to infinity.

This final series representation elegantly captures the essence of the sine function as an infinite polynomial. It is important to note that this series converges for all real values of x, meaning it provides an accurate representation of sin(x) across its entire domain. The alternating signs and increasing factorials in the denominator ensure that the series remains well-behaved, even for large values of x.

Deriving the concepts that underpin the Maclaurin series offers a solid theoretical grounding, the true magic unfolds when we apply this knowledge to a specific function. Let’s now turn our attention to the sine function, sin(x), and embark on a step-by-step journey to derive its Maclaurin series representation. This process will not only illuminate the power of the Maclaurin series but also provide a tangible example of how calculus can be used to express complex functions in a surprisingly elegant form.

Convergence and Representation: Understanding the Series' Behavior

Having derived the Maclaurin series for sin(x), a natural question arises: what does this series truly tell us about the sine function itself? The answer lies in understanding the concepts of convergence and how the power series acts as a representation of sin(x).

The Convergence of the Infinite Series

The Maclaurin series we've constructed is an infinite sum. An infinite sum only holds meaning if it converges to a finite value. Otherwise, the series diverges, rendering it useless as a representation of a function.

Radius and Interval of Convergence

To determine the convergence of a power series, we often examine its radius and interval of convergence. The radius of convergence, denoted by 'R', defines the range around the center of the series (x = 0 for Maclaurin series) within which the series converges. The interval of convergence is the specific interval (e.g., -R < x < R) for which the series yields a finite sum.

For the Maclaurin series of sin(x), a remarkable property holds: the series converges for all real numbers. This means that regardless of the value of 'x' you input into the series, the resulting infinite sum will always converge to a finite value.

This is often expressed as the interval of convergence being (-∞, ∞) and the radius of convergence being infinite (R = ∞). This is a powerful statement about the series' ability to accurately represent the sine function.

Power Series Representation of Sin(x)

The convergence of the Maclaurin series across the entire real number line has a profound implication. It indicates that the power series we derived is a valid representation of the sine function.

Representing the Function Over Its Entire Domain

In essence, we've shown that the infinitely long polynomial expressed by the Maclaurin series perfectly mimics the behavior of sin(x) for every possible value of x.

This means that at any point on the x-axis, the value obtained from the power series will be identical to the value of sin(x). This equivalence holds true across its entire domain.

This underscores the inherent beauty of the Maclaurin series. A seemingly complex trigonometric function, like sin(x), can be perfectly replicated by an infinite sum of simple polynomial terms. This opens doors to powerful approximations and analytical manipulations that would be far more difficult, if not impossible, with the original trigonometric form.

Having explored the fascinating realm of convergence and how the Maclaurin series beautifully mirrors the behavior of the sine function across its entire domain, it's time to pivot towards the practical implications of this mathematical representation. The true value of the sin(x) Maclaurin series lies not just in its theoretical elegance but in its tangible ability to simplify complex calculations and model real-world phenomena.

Practical Applications: Unleashing the Power of the Sin(x) Series

The Maclaurin series representation of sin(x) isn't merely a mathematical curiosity; it's a powerful tool with wide-ranging applications. From approximating values to simplifying complex physical models, this series provides a versatile approach to tackling problems involving the sine function.

Approximating Sin(x): A Practical Guide

One of the most immediate applications of the Maclaurin series is approximating the value of sin(x). By using only the first few terms of the series, we can obtain surprisingly accurate estimations, especially for small values of x. This is particularly useful in situations where direct computation of the sine function is difficult or computationally expensive.

Calculating Sin(x) for Small Values

Let's consider the first few terms of the Maclaurin series for sin(x):

sin(x) ≈ x - (x³/3!) + (x⁵/5!) - ...

For small values of x (close to 0), the higher-order terms (x³, x⁵, etc.) become increasingly smaller, and their contribution to the overall sum diminishes rapidly. Thus, we can often achieve a reasonable approximation by considering only the first term:

sin(x) ≈ x

For example, let's approximate sin(0.1) using just the first term:

sin(0.1) ≈ 0.1

This simple approximation yields a value very close to the actual value of sin(0.1), which is approximately 0.09983.

By adding more terms, we can further improve the accuracy. Using the first two terms:

sin(0.1) ≈ 0.1 - (0.1³/3!) = 0.1 - (0.001/6) ≈ 0.099833

This approximation is even closer to the true value, demonstrating the power of the Maclaurin series in providing accurate estimations.

The Dance of Accuracy: More Terms, Better Approximation

The beauty of the Maclaurin series lies in its ability to provide increasingly accurate approximations as more terms are included. Each additional term refines the estimation, bringing it closer to the true value of the sine function.

Visualizing the Improvement

Imagine a graph plotting the approximation of sin(x) using different numbers of terms from its Maclaurin series.

Initially, with only the first term (sin(x) ≈ x), the approximation is a straight line, accurate only very close to x = 0.

As we add the cubic term (-x³/3!), the approximation begins to curve, more closely resembling the sine wave.

Adding the next term (x⁵/5!) further refines the curve, extending the range over which the approximation is accurate.

This process continues indefinitely, with each additional term improving the approximation and extending its valid range.

Quantifying the Improvement

We can quantify this improvement by calculating the error between the approximation and the true value of sin(x) for different numbers of terms. As more terms are added, the error decreases, demonstrating the increasing accuracy of the approximation.

This concept is crucial in numerical analysis and computer science, where approximations are often used to simplify calculations and reduce computational cost.

Real-World Applications: From Pendulums to Signals

The sin(x) Maclaurin series finds applications in various fields, particularly in physics and engineering, where the sine function plays a central role in modeling oscillatory phenomena.

Pendulum Motion: A Classic Example

One classic example is the analysis of pendulum motion. For small angles, the sine of the angle of displacement can be accurately approximated by the angle itself (sin(θ) ≈ θ). This simplification allows us to model the pendulum's motion using a simple harmonic oscillator equation, which is much easier to solve than the full nonlinear equation.

The simple harmonic oscillator approximation relies on using only the first term of the Maclaurin series for sin(x).

Signal Processing: Deconstructing Waves

In signal processing, the sine function is fundamental to representing and analyzing periodic signals. The Maclaurin series can be used to approximate these signals, allowing for efficient computation and manipulation. For instance, in audio processing, the Maclaurin series can be used to approximate complex waveforms, enabling techniques like filtering and compression.

By representing signals as sums of simpler polynomial terms, engineers can develop efficient algorithms for analyzing and manipulating these signals. This has profound implications in areas such as telecommunications, audio engineering, and medical imaging.

Other Applications

The sin(x) Maclaurin series finds applications in:

• Optics: Approximating diffraction patterns.
• Electromagnetism: Analyzing electromagnetic waves.
• Control Systems: Modeling oscillatory behavior in feedback systems.

In conclusion, the Maclaurin series representation of sin(x) is a versatile tool with a wide range of practical applications. Its ability to provide accurate approximations simplifies complex calculations and enables the modeling of real-world phenomena in various fields. By understanding and harnessing the power of this series, we can gain deeper insights into the behavior of the sine function and its role in the world around us.

Video: Sin(x) Maclaurin Series: The Secret You Need to Know!

Play Video

So, there you have it! Hopefully, this exploration of the sinx maclaurin series was helpful. Go forth and use this knowledge wisely – and maybe impress your friends with your newfound calculus skills!