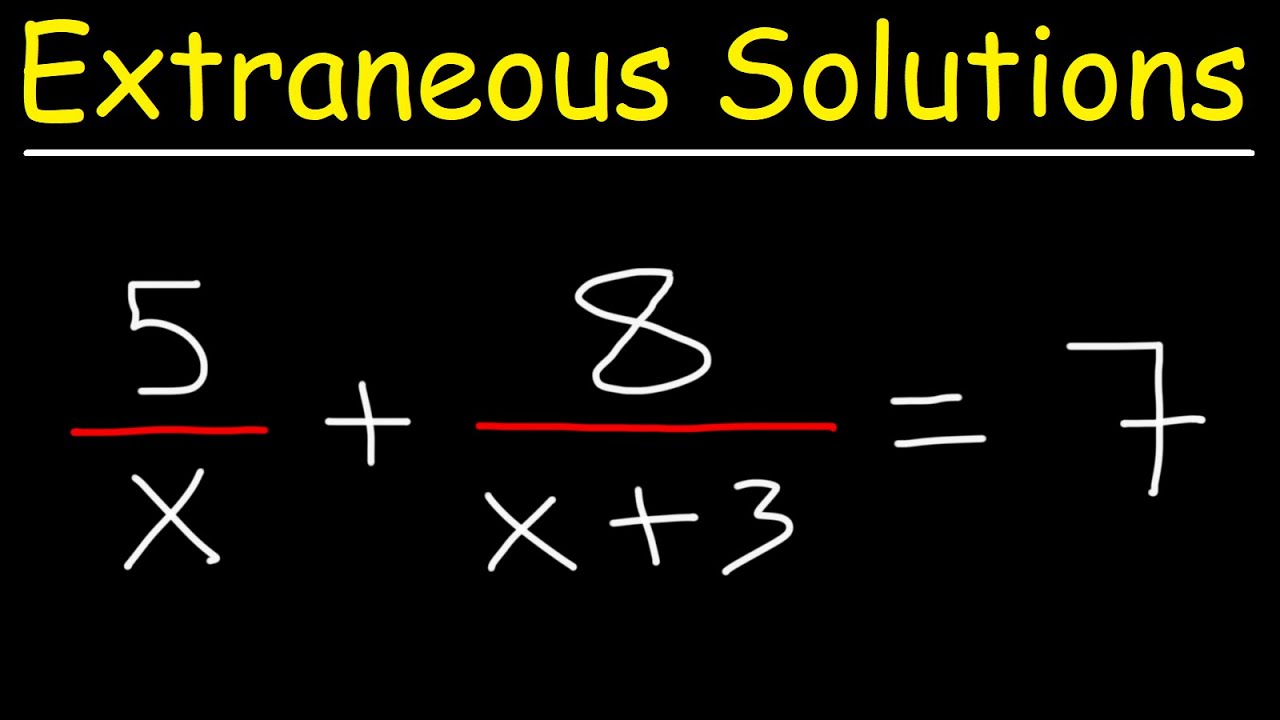Extraneous Solution: Algebra Guide & How to Avoid

Extraneous solutions often puzzle students learning about algebra, arising particularly when dealing with radical equations and rational expressions. One effective strategy to avoid these solutions involves meticulous checking of answers, mirroring techniques advocated by math educators at institutions like the Khan Academy. The core issue lies in the mathematical operations performed during the solving process, which can sometimes introduce values that do not satisfy the original equation, thus leading to an extraneous solution.

Image taken from the YouTube channel The Organic Chemistry Tutor , from the video titled Checking For Extraneous Solutions of Radical Equations .

Algebra, the cornerstone of mathematical understanding, is often perceived as a realm of precise calculations and definitive answers. However, lurking within its elegant equations are subtle traps that can ensnare even the most diligent problem-solvers: extraneous solutions.

These deceptive results, born from legitimate algebraic manipulations, can lead you astray if you're not careful. Let's explore this concept.

What are Extraneous Solutions?

At their core, extraneous solutions are values that emerge as potential solutions during the equation-solving process, yet fail to satisfy the original equation when plugged back in. They are algebraic mirages, appearing valid at first glance but ultimately proving false upon closer inspection.

Think of them like this: you follow a recipe perfectly, but the final dish tastes nothing like what you expected. Where did things go wrong?

The Root of the Problem

These "false positives" typically arise from operations that alter the equation's domain, or introduce new potential solutions that weren't there initially. Squaring both sides of an equation, for instance, can be a primary culprit, as we'll see later.

These types of operations don't necessarily invalidate the solution process, but rather require an additional step: verification. This is the critical step that separates a confident algebraist from one who gets tricked by their math!

Why are Extraneous Solutions Important?

The importance of recognizing and identifying extraneous solutions cannot be overstated. Failing to do so directly leads to incorrect answers, undermining the entire problem-solving effort.

In essence, it renders your calculations meaningless. It is like investing your time into solving the problem with no returns.

Moreover, the ability to detect these false solutions demonstrates a deeper understanding of algebraic principles and a commitment to precision. This ensures that you are not simply applying rules blindly, but rather thinking critically about the nature of the problem.

Core Misconception: The Illusion of Validity

A common mistake among students is the assumption that every solution obtained through algebraic manipulation is automatically valid. It's tempting to trust the process and accept the final result without question. However, algebra demands skepticism.

The most crucial rule in problem-solving (especially when dealing with radicals, rationals or absolute values) is to always verify your answers by substituting them back into the original equation. Doing so is a necessity, not an option.

Challenge every solution! This is the only way to avoid being fooled by these deceptive algebraic interlopers.

Common Culprits: Sources of Extraneous Solutions

Algebra, the cornerstone of mathematical understanding, is often perceived as a realm of precise calculations and definitive answers. However, lurking within its elegant equations are subtle traps that can ensnare even the most diligent problem-solvers: extraneous solutions. These deceptive results, born from legitimate algebraic manipulations, can lead you astray if you're not careful. Let's explore the equation types most susceptible to these mathematical mirages and the algebraic steps that often pave their way.

Radical Equations: When Squaring Creates Ghosts

Radical equations, those containing radicals (like square roots, cube roots, and so on), are notorious for producing extraneous solutions. This stems from the operations we often use to solve them, particularly squaring both sides of the equation.

The Peril of Squaring

Squaring both sides is a common technique to eliminate radicals. However, it's a non-reversible operation that can introduce solutions that don't work in the original equation. Why? Because squaring masks the sign. It turns both positive and negative numbers into positive ones.

For instance, consider the equation √(x) = -3.

Intuitively, we know there's no real number whose square root is negative.

However, if we square both sides, we get x = 9.

But, wait! If we substitute x = 9 back into the original equation, we get √(9) = 3, not -3. So, x = 9 is an extraneous solution. The equation √(x) = -3 has no real solution.

Example Walkthrough: Unmasking the Extraneous

Let's examine the equation √(x+3) = x-3. Here's how extraneous solutions can creep in:

1. Square both sides: This yields (x+3) = (x-3)², which simplifies to x+3 = x² - 6x + 9.
2. Rearrange into a quadratic: x² - 7x + 6 = 0.
3. Factor: (x-6)(x-1) = 0.
4. Potential solutions: x = 6 or x = 1.

Now, let's check:

• x = 6: √(6+3) = √(9) = 3, and 6-3 = 3. So, x = 6 is a valid solution.
• x = 1: √(1+3) = √(4) = 2, and 1-3 = -2. So, x = 1 is an extraneous solution because 2 ≠ -2.

Therefore, the only valid solution to √(x+3) = x-3 is x = 6. The value x = 1 appeared during the solving process. Yet it does not satisfy the original equation. Always check to find the actual solution.

Rational Equations: Beware of the Denominator's Revenge

Rational equations, involving fractions with variables in the denominator, are another frequent source of extraneous solutions. The culprit here is often multiplying both sides of the equation by an expression containing a variable.

Multiplying by Variable Expressions: A Risky Move

This is a common technique to clear denominators. However, it can introduce solutions that make the original denominator equal to zero, which is undefined. Remember, division by zero is a big no-no in mathematics.

These "solutions" are extraneous because they violate the domain of the original equation. That is, they are not valid values for 'x' that the equation allows.

Example Walkthrough: Zeroing Out the Denominator

Consider the equation x/(x-2) = 2/(x-2).

1. Multiply both sides by (x-2): This gives x = 2.

2. Check: Substituting x = 2 back into the original equation results in 2/(2-2) = 2/0. This is undefined.

Therefore, x = 2 is an extraneous solution. The equation x/(x-2) = 2/(x-2) has no solution.

Absolute Value Equations: A Tale of Two Paths

Absolute value equations, dealing with the absolute value of expressions, can also lead to extraneous solutions. Similar to radical equations, the issue arises when we square both sides (after isolating the absolute value) to eliminate the absolute value signs.

Squaring Absolute Values: A Double-Edged Sword

Squaring both sides can introduce extraneous solutions because the absolute value makes an expression non-negative. Squaring it removes the sign information, potentially creating solutions that don't hold true in the original equation.

Example Walkthrough: Absolute Value Ambiguity

Let’s consider the equation |2x + 1| = 3x + 4

1. Because of the absolute value, we have two scenarios to consider:

   • Scenario 1: 2x + 1 = 3x + 4, which leads to x = -3
   • Scenario 2: 2x + 1 = -(3x + 4), which leads to x = -1

2. Check both potential solutions:

   • For x = -3, |2(-3) + 1| = |-5| = 5, whereas 3(-3) + 4 = -5. Since 5 ≠ -5, x = -3 is an extraneous solution.
   • For x = -1, |2(-1) + 1| = |-1| = 1, whereas 3(-1) + 4 = 1. Since 1 = 1, x = -1 is a valid solution.

In this case, after checking potential solutions, only x = -1 is a valid solution.

The Detective Work: Solving Equations and Spotting Extraneous Solutions

After identifying the usual suspects—radical, rational, and absolute value equations—it's time to put on our detective hats and delve into the process of solving these equations while carefully watching for extraneous solutions. It's a meticulous procedure, but with a systematic approach and a keen eye, you can navigate these algebraic puzzles successfully.

Solving Equations: A Methodical Approach

The key to solving equations prone to extraneous solutions lies in a structured, step-by-step method. This helps minimize errors and makes it easier to spot potential red flags.

Step 1: Isolation is Key

Begin by isolating the radical or absolute value term, if present. This means getting the radical or absolute value expression by itself on one side of the equation. This step simplifies subsequent operations.

For example, if you have √(x + 2) + 3 = 5, first subtract 3 from both sides to get √(x + 2) = 2.

Step 2: Perform Necessary Operations

Next, perform the necessary operations to eliminate the radical or absolute value. This typically involves squaring (for square roots), cubing (for cube roots), or multiplying to clear denominators in rational equations.

Remember: This is where extraneous solutions often creep in. Squaring both sides, for instance, can introduce solutions that don't satisfy the original equation because it disregards the sign.

Step 3: Solve the Resulting Equation

After eliminating the radical or absolute value, you'll be left with a simpler equation. Solve this equation using standard algebraic techniques. This might involve factoring, using the quadratic formula, or simply isolating the variable.

Simplification: Accuracy Matters

Throughout the solving process, meticulous simplification is paramount. Careless mistakes in simplification can easily lead to incorrect solutions, which then become difficult to identify as extraneous.

Double-checking each step, especially when dealing with exponents and fractions, is crucial. A small error early on can snowball into a misleading answer.

Think of it like building a house – a faulty foundation will compromise the entire structure.

Verification: The Ultimate Test

The most critical step in identifying extraneous solutions is verification. This involves substituting each potential solution back into the original equation.

Never substitute into a modified version of the equation, as this won't reveal extraneous solutions introduced during the solving process.

If the solution satisfies the original equation, it's a valid solution. If it doesn't, it's an extraneous solution, and you must discard it.

Verification is not optional – it's the final safeguard against incorrect answers. It's the algebraic equivalent of a detective confirming their suspect's alibi. Without it, you can't be sure you've solved the case correctly.

Behind the Scenes: Understanding Domain and Range

[The Detective Work: Solving Equations and Spotting Extraneous Solutions After identifying the usual suspects—radical, rational, and absolute value equations—it's time to put on our detective hats and delve into the process of solving these equations while carefully watching for extraneous solutions. It's a meticulous procedure, but with a systemati...]

To truly master the art of identifying extraneous solutions, we must venture behind the scenes and explore the fundamental concepts of domain and range. These aren't just abstract mathematical ideas; they are the gatekeepers that determine which solutions are valid and which are imposters.

Understanding domain and range is like having a secret weapon in your algebraic arsenal. Let's unlock its power.

The Unyielding Importance of the Domain

The domain of an equation is the set of all possible input values (usually 'x') for which the equation is defined. Think of it as the equation's "comfort zone." Extraneous solutions often trespass beyond this zone, violating the equation's fundamental restrictions.

It is the collection of values for which the function is defined.

Why is understanding the domain so important?

Because extraneous solutions frequently arise when we perform operations that alter the original domain.

Common Domain Restrictions: A Checklist

Here's a quick checklist of common domain restrictions to keep in mind:

• Denominators: Denominators in rational expressions cannot be zero. Any solution that makes a denominator zero is automatically extraneous.

  It is very easy to introduce mistakes when working with rational equations.

• Radicals (Even Roots): Expressions under even roots (like square roots, fourth roots, etc.) cannot be negative. Solutions that lead to negative values under an even root are extraneous.

  Remember to isolate the radical before squaring.

• Logarithms: The argument of a logarithm must be positive. This is less common in basic algebra, but crucial to consider in more advanced mathematics.

  Note that the base of the logarithm cannot be equal to one.

Domain Violations: Examples

Let's illustrate this with an example:

Consider the equation √(x - 2) = 5. The domain restriction is x ≥ 2 because the expression under the square root must be non-negative. If, through some algebraic error, we arrived at a solution where x < 2, we would immediately know it's extraneous.

Relevance of the Range: A Subtle Guide

The range of an equation is the set of all possible output values (usually 'y'). While less directly tied to extraneous solutions than the domain, understanding the range can still offer valuable clues.

The range is the set of possible output values after you have substituted the domain.

It tells you about the overall values you can get.

Range Considerations: A Conceptual Tool

Think of the range as a guide rather than a strict rule.

If you know that a particular equation cannot produce negative values (for example, the square of a real number), and a solution leads to a negative output, it's a strong indication that something went wrong.

This might not definitively identify an extraneous solution, but it raises a red flag, prompting you to double-check your work.

How Range Helps

Here's how understanding range plays a role in identifying inconsistencies:

Suppose we solve an equation and find that y = -5, but, after analyzing the equation, we know that the function can only yield positive numbers because of its definition. Then, there must be a mistake somewhere in our calculations.

Understanding the range will help us identify this mistake.

By keeping domain and range in mind, we not only avoid extraneous solutions but also deepen our understanding of how equations behave, leading to more confident and accurate problem-solving.

Extraneous Solutions in Context: Different Types of Equations

After identifying the usual suspects—radical, rational, and absolute value equations—it's time to put on our detective hats and delve into the process of solving these equations while carefully watching for extraneous solutions. Now, let's consider how this concept extends to other corners of the algebraic landscape.

Quadratic Equations and Extraneous Solutions: A Nuanced View

The world of quadratic equations, with its parabolas and potential for two solutions, introduces a subtle, yet crucial, element to our understanding of extraneous solutions.

While the mechanics of solving a quadratic equation generally don't introduce extraneous roots the same way squaring a radical equation does, the context in which the equation is presented can certainly make a solution extraneous.

Context, context, context: the single most determining factor for extraneous solutions in quadratic equations.

Consider word problems, for instance.

Perhaps you're modeling the dimensions of a rectangle, where x represents the width. If solving the quadratic yields x = -5 and x = 3, the negative solution is clearly extraneous because dimensions cannot be negative.

Pay close attention to the constraints or conditions imposed by the original problem. This is where valid algebraic solutions meet the real-world test.

Think about the physical limitations of a situation or any explicitly stated restrictions on the variable. Overlooking these aspects is a common pitfall.

Inequalities: A Glimpse Beyond Equations

While the primary focus of extraneous solutions lies within the realm of equations, the underlying principles can, to some extent, be applied to inequalities.

The key difference is that with inequalities, we are dealing with a range of possible values rather than specific, discrete solutions.

In cases where operations are performed on inequalities that could alter the solution set (such as multiplying by a negative number, which requires flipping the inequality sign), it is essential to consider whether the resulting solution set aligns with the original inequality's domain and any contextual constraints.

Although the term "extraneous solution" is not commonly used, the idea of a solution that doesn't hold true within the original inequality's restrictions certainly resonates.

The focus shifts from checking individual values to ensuring that the entire solution interval remains valid.

Your Toolkit: Resources for Identifying and Avoiding Extraneous Solutions

Extraneous solutions can feel like sneaky saboteurs, but thankfully, you don't have to face them unarmed! A wealth of resources are available to assist in identifying and avoiding these algebraic traps. Let's explore some of the most helpful tools and how to leverage them effectively.

Visualizing Solutions with Graphing Calculators

Graphing calculators, such as the TI-84, and online platforms like Desmos and GeoGebra, offer a powerful visual aid in understanding equations and their solutions.

By plotting the equation, you can visually identify discontinuities or points where the equation is undefined.

These points often signal potential extraneous solutions.

Moreover, you can trace the graph to see where it intersects the x-axis, representing the real solutions. If an algebraically derived solution doesn't appear on the graph, it's a strong indication that it's extraneous.

Harnessing the Power of Computer Algebra Systems

For more complex equations, Computer Algebra Systems (CAS) like Mathematica, Maple, and SageMath can be invaluable. These systems not only solve equations but also verify the solutions, saving you time and effort.

CAS can handle symbolic manipulation and complex calculations with ease. This makes them useful for tackling equations that are difficult or impossible to solve by hand.

Remember that while CAS are powerful, they should be used to supplement your understanding, not replace it entirely.

Building a Solid Foundation with Textbooks

Oldies but goodies! Algebra 1, Algebra 2, and Precalculus textbooks provide a thorough foundation in the underlying concepts necessary to understand and solve equations.

They offer detailed explanations, worked examples, and plenty of practice problems. Don't underestimate the value of working through textbook problems.

This will help you develop a deeper understanding of the material. Look for sections that specifically address extraneous solutions and provide strategies for identifying them.

Exploring Online Math Resources

The internet is a treasure trove of math resources! Platforms like Khan Academy, Mathway, and Wolfram Alpha offer explanations, examples, and even equation solvers.

Khan Academy provides free video lessons and practice exercises that cover a wide range of algebra topics. Mathway and Wolfram Alpha can solve equations step-by-step, allowing you to see the solution process in detail.

These resources can be especially helpful when you're stuck on a problem or need additional practice. Use them to reinforce your understanding and build confidence.

Leveraging Educational Websites and Platforms

Beyond the general math resources, many websites and platforms are specifically dedicated to algebra education.

These platforms often offer interactive lessons, quizzes, and personalized feedback.

Look for resources that focus on problem-solving strategies and common mistakes. Engaging with these resources can make learning algebra more effective and enjoyable.

By utilizing these resources effectively, you can build a robust toolkit for identifying and avoiding extraneous solutions, ultimately becoming a more confident and successful algebra student.

Avoiding the Pitfalls: Common Mistakes and Misconceptions

[Your Toolkit: Resources for Identifying and Avoiding Extraneous Solutions Extraneous solutions can feel like sneaky saboteurs, but thankfully, you don't have to face them unarmed! A wealth of resources are available to assist in identifying and avoiding these algebraic traps. Let's explore some of the most helpful tools and how to leverage them eff...]

Navigating the world of extraneous solutions requires more than just algebraic skills. It demands a clear understanding of the underlying concepts and a sharp eye for common errors. One of the most frequent stumbling blocks is the confusion between extraneous solutions and equations that have "no solution."

Let's break down this critical distinction.

Extraneous Solutions vs. No Solution: Knowing the Difference

It's easy to conflate these two scenarios, but they represent fundamentally different outcomes. An extraneous solution is a value that emerges during the solving process but doesn't actually satisfy the original equation. It's a false positive, a mathematical mirage.

"No solution," on the other hand, indicates that there is no value that, when substituted into the original equation, will make the equation true. The equation is inherently contradictory, regardless of your solving efforts.

Extraneous Solutions: A Result of the Process

Extraneous solutions often arise from operations that aren't reversible in the strictest sense. Squaring both sides of an equation is a prime example.

Recall our discussion of radical equations. When you square both sides of an equation like √(x) = -3, you get x = 9. However, substituting x = 9 back into the original equation yields √9 = -3, which simplifies to 3 = -3. This is clearly false, making x = 9 an extraneous solution.

The squaring process introduced a potential solution that didn't exist in the initial equation's domain. The initial equation stated "the square root of x must be -3", which is impossible in the real number system (the square root is only positive).

"No Solution": An Inherent Contradiction

In contrast, consider an equation like |x| = -5. The absolute value of any number is always non-negative. Thus, there is no real number that, when plugged into the absolute value, will equal -5. The equation is fundamentally impossible.

There is no algebraic manipulation that can change this fact. You can't "solve" it to find a valid solution because there isn't one.

Spotting the Difference: A Crucial Skill

The key to distinguishing between these scenarios lies in careful verification.

Always substitute your potential solutions back into the original equation. If the equation holds true, the solution is valid. If it leads to a contradiction, it's extraneous.

If, after attempting to solve an equation using standard algebraic methods, you arrive at a contradiction (e.g., 0 = 1) before finding a potential value for the variable, then the equation has "no solution."

Think of it this way:

• Extraneous Solution: You find a potential solution, but it's a fake.
• No Solution: You can't find any solution, and the equation itself is the problem.

Understanding this difference is vital for accurate problem-solving. It prevents you from incorrectly accepting extraneous solutions and helps you recognize when an equation is simply unsolvable.

Video: Extraneous Solution: Algebra Guide & How to Avoid

Play Video

So, next time you're knee-deep in algebraic equations, remember that extraneous solution lurks! Double-checking your answers might seem tedious, but it's a lifesaver. Happy solving!