Vertical vs Horizontal Dilation: Real Examples

The transformative geometry represented by vertical dilation vs horizontal dilation manifests alterations in a function's graph, impacting its spatial dimensions. Specifically, vertical dilation vs horizontal dilation can be visually demonstrated using tools like Desmos, where functions undergo transformations affecting their height or width. These concepts are crucial in fields such as seismology, where the analysis of seismic waves involves understanding how signals stretch or compress along different axes. Moreover, the implications of vertical and horizontal dilation are deeply explored within the frameworks established by mathematicians like Euclid, whose foundational work on geometry provides the basis for understanding these transformations.

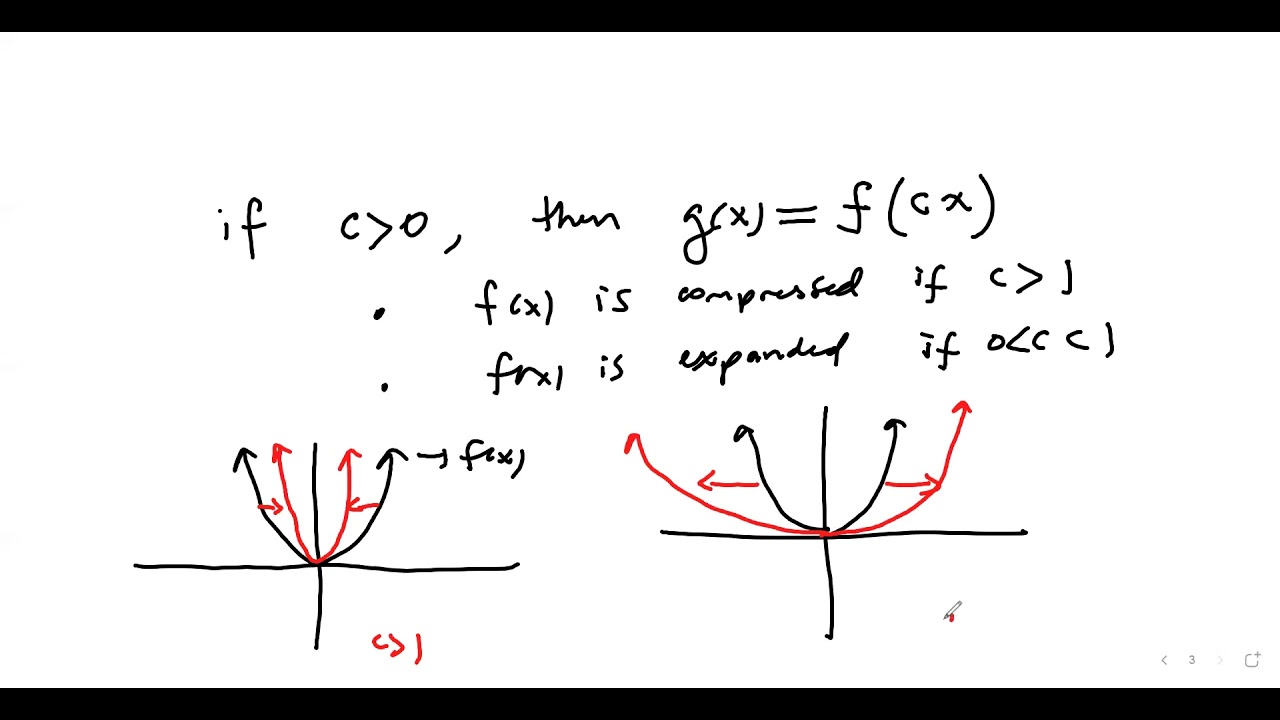
Image taken from the YouTube channel MyLearnMate , from the video titled Vertical and Horizontal Dilation .

Dilation, at its core, is a mathematical transformation that alters the size of an object without changing its shape. It's a fundamental concept that underpins a multitude of applications, from the seemingly simple act of resizing an image to complex scientific modeling. Understanding dilation provides crucial insights into scaling transformations.

In precise mathematical terms, dilation is a transformation that produces an image that is the same shape as the original, but is a different size. A dilation is defined by two key elements: a center of dilation and a scale factor. The center acts as a fixed point around which the object expands or contracts, while the scale factor determines the magnitude of the size change.

The Significance of Dilation: Why It Matters

The significance of dilation extends far beyond abstract mathematical exercises. Dilation plays a vital role in various disciplines, providing a framework for understanding and manipulating scale.

Consider its use in cartography, where maps are, in essence, dilations of geographical regions. Or, consider architecture, where blueprints are scaled-down representations of buildings. From the creation of detailed medical images to the development of immersive video game environments, dilation proves to be an indispensable tool.

Its applications span various fields, impacting how we visualize, design, and interact with the world around us.

Navigating this Exploration of Dilation

This exploration will delve into the mathematical foundations of dilation, clarifying the roles of the coordinate plane and scale factors. We will explore its relationship with other transformations and its impact on functions.

Furthermore, we will showcase real-world applications ranging from image editing to economic data visualization. Finally, we will present some of the most useful tools and technologies for exploring dilation.

Mathematical Foundations of Dilation: The Coordinate Plane and Scale Factor

Dilation, at its core, is a mathematical transformation that alters the size of an object without changing its shape. It's a fundamental concept that underpins a multitude of applications, from the seemingly simple act of resizing an image to complex scientific modeling. Understanding dilation provides crucial insights into scaling transformations, and this section will focus on the coordinate plane and scale factor to provide an essential mathematical foundation.

Dilation in the Coordinate Plane

Dilation is readily visualized and mathematically defined within the coordinate plane. Here, points are transformed relative to a fixed point known as the center of dilation. Understanding how dilation operates in this environment is key to grasping its properties.

When a figure undergoes dilation, each of its points moves either closer to or further away from the center of dilation. The new position of each point is determined by a scale factor, which dictates the magnitude of the change in distance.

The Center of Dilation: Origin and Beyond

The center of dilation serves as the anchor point for the transformation. While the origin (0, 0) is often used for simplicity, the center can, in fact, be any point on the coordinate plane.

When the origin is the center, the coordinates of a point (x, y) after dilation with a scale factor k become (kx, ky). This means each coordinate is simply multiplied by the scale factor.

However, when the center of dilation is not the origin, the transformation requires a more complex calculation. One must first translate the figure so that the center of dilation coincides with the origin. Next, perform the dilation as described above, and finally, translate the figure back to its original position.

This process, although slightly more involved, ensures accurate scaling relative to the chosen center.

The Scale Factor: Defining Enlargement and Reduction

The scale factor is the cornerstone of dilation. It's a numerical value that determines how much larger or smaller the image becomes after the transformation. The scale factor dictates whether the dilation will result in an enlargement or a reduction of the original figure.

• Enlargement: If the scale factor k is greater than 1 (k > 1), the resulting image will be larger than the original. Each point moves further away from the center of dilation. The distances between points increase proportionally.

• Reduction: Conversely, if the scale factor k is between 0 and 1 (0 < k < 1), the resulting image will be smaller than the original. Each point moves closer to the center of dilation. The distances between points decrease proportionally.

• Scale Factor of 1: If the scale factor k is exactly 1 (k = 1), the dilation results in no change. The image is congruent to the original, and no transformation occurs.

• Negative Scale Factor: When the scale factor is negative, the dilation includes a rotation of 180 degrees about the center of dilation, in addition to scaling. The absolute value of the scale factor determines the size change, while the negative sign indicates the rotation.

The scale factor is thus a powerful tool. It provides precise control over the size and orientation of the dilated image. By understanding its properties, one can accurately predict and manipulate the outcome of dilation transformations.

Dilation and Other Transformations: Linear Transformations and Stretching/Compression

Dilation, at its core, is a mathematical transformation that alters the size of an object without changing its shape. It's a fundamental concept that underpins a multitude of applications, from the seemingly simple act of resizing an image to complex scientific modeling. Understanding where dilation fits within the broader landscape of geometric transformations provides a richer and more nuanced appreciation of its power.

Dilation as a Linear Transformation

Dilation isn't an isolated concept.

It's best understood as a specific type of linear transformation.

Linear transformations are operations that preserve vector addition and scalar multiplication.

In simpler terms, they maintain the linear relationships between points.

Dilation adheres to these properties, ensuring that lines remain lines and parallel lines remain parallel after the transformation.

This characteristic distinguishes it from non-linear transformations, which can distort shapes and alter these fundamental relationships.

Connecting Dilation to Stretching and Compression

The concept of stretching and compression is inextricably linked to dilation.

When the scale factor in a dilation is greater than 1, the object undergoes stretching or enlargement.

Every point moves further away from the center of dilation.

Conversely, when the scale factor is between 0 and 1, the object experiences compression or reduction.

In this case, every point moves closer to the center of dilation.

It's crucial to recognize that stretching and compression are directional; they occur along lines emanating from the center of dilation.

The scale factor determines the magnitude of this stretching or compression.

Dilation's Role in the Family of Transformations

Dilation is a member of a larger family of transformations that include translations, rotations, and reflections.

While these other transformations focus on repositioning or mirroring objects, dilation uniquely addresses size modification.

It is also important to note that dilation is often combined with other transformations.

For instance, an object could be rotated and then dilated, or translated and then compressed.

The order in which these transformations are applied matters and can yield different final results. Understanding the individual effects of each transformation, including dilation, is key to predicting and controlling the outcome of complex sequences of transformations. By considering how dilation fits into this broader context, we gain a more comprehensive view of its role and utility in mathematics and its myriad applications.

Dilation and Functions: Visualizing Transformations on Graphs

Dilation, at its core, is a mathematical transformation that alters the size of an object without changing its shape. It's a fundamental concept that underpins a multitude of applications, from the seemingly simple act of resizing an image to complex scientific models. In this section, we explore how dilation impacts the graphical representation of functions, providing visual insights into these transformations.

Understanding Dilation's Effect on Function Graphs

When applied to functions, dilation can significantly alter their appearance on a graph. The key is to understand how the scale factor influences the coordinates of points on the function. Dilation essentially stretches or compresses the graph either vertically or horizontally, depending on how the transformation is applied.

Vertical vs. Horizontal Dilation: A Comparative Analysis

The effect of dilation on a function's graph depends on whether it's applied vertically or horizontally. These two types of dilation exhibit distinct characteristics and impact the graph in different ways.

Vertical Dilation: Stretching and Compressing Along the Y-Axis

Vertical dilation involves multiplying the function's output (y-value) by the scale factor.

A scale factor greater than 1 stretches the graph vertically, making it taller. Conversely, a scale factor between 0 and 1 compresses the graph vertically, making it shorter.

For example, if we have the function f(x) and we apply a vertical dilation with a scale factor of k, the new function becomes k f(x). This transformation changes the y-values of each point on the graph while leaving the x-values unchanged.

Horizontal Dilation: Modifying the Input Values

Horizontal dilation, on the other hand, involves multiplying the function's input (x-value) by the reciprocal of the scale factor.

A scale factor greater than 1 compresses the graph horizontally, making it narrower. A scale factor between 0 and 1 stretches the graph horizontally, making it wider.

If we apply a horizontal dilation with a scale factor of k to the function f(x), the new function becomes f(x/k). This transformation alters the x-values of each point on the graph, affecting its horizontal spread.

Visualizing Dilation with Graphing Calculators: Desmos and TI-84

Graphing calculators are invaluable tools for visualizing how dilation affects function graphs. Platforms like Desmos and TI-84 offer dynamic environments where you can manipulate functions and observe the resulting transformations in real-time.

Desmos: An Intuitive Online Graphing Tool

Desmos is a free, online graphing calculator that's exceptionally user-friendly. It allows you to easily input functions and apply dilation transformations.

You can experiment with different scale factors and observe how the graph stretches or compresses accordingly. Desmos also allows you to define sliders for the scale factors, making it interactive to see these transformations.

TI-84: A Versatile Handheld Calculator

The TI-84 is a popular handheld graphing calculator widely used in education. While it may require some initial setup, it provides a powerful platform for visualizing function transformations.

You can input functions, define variables for the scale factors, and observe how the graph changes as you adjust these variables. Understanding how to use the TI-84 for dilation can greatly enhance your grasp of the concepts.

Interactive Exploration with GeoGebra

GeoGebra is another powerful tool for exploring dilation interactively. It combines geometry, algebra, calculus, and more into a single, easy-to-use platform. With GeoGebra, you can create dynamic geometric constructions that illustrate the effects of dilation on functions.

You can define functions, create sliders for scale factors, and observe how the graph transforms in real-time as you adjust the sliders. This hands-on approach provides a deeper understanding of the underlying mathematical principles. GeoGebra's strength lies in its ability to seamlessly integrate algebraic and geometric representations, providing a holistic view of transformations.

Real-World Applications of Dilation: From Images to Economics

Dilation, at its core, is a mathematical transformation that alters the size of an object without changing its shape. It's a fundamental concept that underpins a multitude of applications, from the seemingly simple act of resizing an image to complex scientific models. In this section, we'll explore the ubiquitous presence of dilation in everyday life, examining its practical implications across various domains.

Dilation in Visual Media and Design

Digital Image Scaling

Image editing software like Photoshop and GIMP heavily rely on dilation algorithms. When you resize an image, you're essentially performing a dilation.

The software uses sophisticated algorithms to maintain image quality while enlarging or reducing the number of pixels. Without these, images would become pixelated and distorted.

Cartography and Architectural Design

Maps are prime examples of dilation in action. They are scaled-down representations of geographical areas, allowing us to visualize vast landscapes on a manageable surface.

Similarly, architectural blueprints are scaled representations of structures, allowing architects and engineers to plan and execute construction projects with precision.

The scale factor is crucial here, ensuring that proportions are maintained while significantly reducing the physical size.

Dilation in Science and Technology

Optical Instruments

Microscopes and telescopes utilize lenses to create dilated images of small or distant objects. These instruments use carefully crafted lenses to magnify the subject, enabling scientists and researchers to observe details that would otherwise be invisible.

The magnification power of these instruments directly depends on the dilation factor achieved by the lens system.

Reproduction Technology

Photocopiers and printers also employ dilation to enlarge or reduce documents. This process requires precise control over the scaling factor to ensure accurate reproduction.

Whether it's enlarging a small document for better readability or reducing a large document to fit a specific format, dilation plays a critical role in the reproduction process.

Dilation in the Digital World

Video Game Design

Video games use dilation principles extensively for scaling objects. As players move through virtual environments, objects in the distance are scaled down to create the illusion of depth and perspective.

When a character grows or shrinks, dilation principles change in the game environment.

These scaling operations are performed in real-time, requiring efficient algorithms to maintain smooth gameplay.

Audio Engineering and Time Manipulation

In audio engineering, time stretching is achieved through horizontal dilation of audio waveforms. This technique allows producers and engineers to adjust the tempo of a song without affecting its pitch.

This process involves compressing or expanding the timeline of the audio signal, effectively changing the duration of sounds.

Dilation in Data Visualization and Analysis

Economic Data Representation

Economic data is often visualized using graphs that can be compressed or stretched to alter the timescale.

This allows analysts to focus on specific periods of interest or to compare trends over different timeframes.

Spreadsheet Software

Spreadsheet software like Microsoft Excel and Google Sheets enables users to create graphs and visualize data that can be easily scaled.

This is particularly useful for analyzing trends, identifying patterns, and presenting information in a clear and concise manner.

The ability to adjust the scale of a graph can reveal insights that might otherwise be missed.

In essence, dilation isn't confined to the realm of pure mathematics. It is a practical and powerful tool that finds application across a wide spectrum of human endeavors. From manipulating images to visualizing economic trends, dilation is a fundamental concept that shapes the way we perceive and interact with the world around us.

Tools and Technologies for Exploring Dilation: Graphing Calculators and Software

[Real-World Applications of Dilation: From Images to Economics Dilation, at its core, is a mathematical transformation that alters the size of an object without changing its shape. It's a fundamental concept that underpins a multitude of applications, from the seemingly simple act of resizing an image to complex scientific models. In this section, we shift our focus to the tools and technologies that empower us to explore dilation, moving from theoretical understanding to practical application.]

Leveraging Technology for Dilation Exploration

The exploration of dilation, once confined to manual geometric constructions, has been revolutionized by readily available technologies. Graphing calculators, dynamic geometry software, and image editing applications now provide powerful platforms for visualizing and manipulating dilations in various contexts. These tools not only simplify complex calculations but also offer interactive environments that foster a deeper intuitive understanding.

Graphing Calculators: Visualizing Function Transformations

Graphing calculators, such as those from the TI-84 series or online platforms like Desmos, are invaluable for visualizing how dilation affects functions. By inputting functions and manipulating parameters, users can observe real-time changes in the graph's shape and position. This is particularly useful for understanding vertical and horizontal dilations.

These calculators allow students to explore the relationship between the scale factor and the resulting transformation. For example, students can easily experiment with multiplying a function by a constant to observe vertical dilation or modifying the input variable to achieve horizontal dilation. This experimentation solidifies their understanding of how algebraic changes translate to geometric transformations.

The ability to dynamically adjust the scale factor and immediately see the corresponding changes on the graph provides a powerful tool for learning and teaching. The calculators help students develop a visual intuition for the concept of dilation that goes beyond rote memorization of formulas.

GeoGebra: Interactive Geometry and Transformations

GeoGebra is a dynamic mathematics software that provides a versatile platform for exploring geometric concepts, including dilation. Its interactive environment allows users to construct geometric figures, define dilations, and observe the resulting transformations in real-time.

Unlike static diagrams, GeoGebra enables users to manipulate the center of dilation, adjust the scale factor, and explore the effects on the geometric figures dynamically. This interactivity is vital for developing a deeper understanding of the properties of dilation.

Furthermore, GeoGebra's scripting capabilities allow users to create custom tools and simulations for specific dilation scenarios. This expands the tool's functionality beyond basic constructions, enabling users to investigate more complex aspects of dilation. Its ability to visually represent the transformation makes abstract mathematical concepts more tangible and accessible.

Image Editing Software: Scaling in the Digital Realm

Image editing software, such as Photoshop and GIMP, offers practical applications of dilation in the context of digital images. These programs allow users to resize images by specifying a scale factor or by manually dragging the corners of the image.

This allows users to see how dilation affects image resolution and quality. Users can observe how enlarging an image beyond a certain point can lead to pixelation, while reducing an image may result in loss of detail.

Moreover, image editing software often provides advanced features like resampling algorithms that optimize the image quality during scaling. By exploring these features, users gain insight into the mathematical techniques used to minimize distortion during dilation. Image editing tools bridge the gap between theoretical mathematics and practical applications, demonstrating how dilation is used to manipulate visual content in the digital world.

Video: Vertical vs Horizontal Dilation: Real Examples

Play Video

So, next time you're resizing an image or tweaking a graph, remember the difference between vertical dilation vs horizontal dilation. A little understanding of how these transformations work can save you a ton of frustration and maybe even unlock some creative possibilities you hadn't considered before!