Master Congruent Arcs: Geometry's Secret Weapon!

Geometry, the cornerstone of spatial reasoning, relies on fundamental principles. Congruent arcs, a key element in this field, directly impact calculations related to circle theorems. Moreover, proficiency with these arcs greatly benefits students studying at institutions such as Khan Academy. The application of congruent arcs principles is facilitated by tools like compass and straightedge for precise constructions. Understanding these concepts allows one to explore advanced applications, which were notably explored by mathematicians, Euclid in his foundational work.

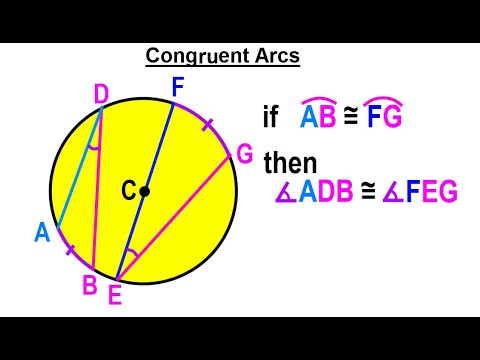
Image taken from the YouTube channel Eat Pi , from the video titled Identify Congruent Arcs | Geometry | Eat Pi .

Geometry, at its core, is the study of shapes, sizes, relative positions of figures, and the properties of space. It's a fundamental branch of mathematics that provides the framework for understanding the world around us, from the structure of buildings to the curves of celestial bodies.

Within the vast landscape of geometry, circles hold a place of particular elegance and utility. And within circles, the concept of congruent arcs emerges as a critical tool for solving a wide range of geometric problems.

Defining Congruent Arcs

So, what exactly are congruent arcs? Simply put, congruent arcs are arcs within the same circle, or within congruent circles, that have equal measures.

Think of it like this: Imagine two slices of the same-sized pizza, cut from the center. If those slices have the same angle at the center, then the crusts of those slices (the arcs) are congruent.

The Significance of Congruent Arcs

The significance of congruent arcs lies in their ability to unlock relationships within circles. They provide a direct link between angles, lengths, and areas, enabling us to deduce information about one part of a circle based on what we know about another.

Understanding congruent arcs is not merely an academic exercise; it's a practical skill with applications in various fields, from engineering to design.

Thesis Statement: Mastering Congruent Arcs for Problem-Solving

This exploration will demonstrate that a comprehensive understanding of congruent arcs is essential for effective problem-solving in geometry. By mastering the properties and theorems associated with congruent arcs, you can unlock a deeper understanding of circles and their applications, and gain a more intuitive approach to geometric problem-solving.

Geometry, at its core, is the study of shapes, sizes, relative positions of figures, and the properties of space. It's a fundamental branch of mathematics that provides the framework for understanding the world around us, from the structure of buildings to the curves of celestial bodies.

Within the vast landscape of geometry, circles hold a place of particular elegance and utility. And within circles, the concept of congruent arcs emerges as a critical tool for solving a wide range of geometric problems.

Foundational Concepts: Circles and Arc Length Explained

Before we can fully appreciate the intricacies of congruent arcs, we must first solidify our understanding of the fundamental building blocks: circles themselves, and the concept of arc length. These are the essential tools in our geometric toolbox.

The Anatomy of a Circle

A circle, in its purest form, is defined as the set of all points in a plane that are equidistant from a single point, which is known as the center.

This seemingly simple definition gives rise to a wealth of properties that make circles both mathematically elegant and practically useful.

• Radius: The distance from the center of the circle to any point on the circle. All radii of the same circle are, by definition, congruent.
• Diameter: A line segment that passes through the center of the circle and has endpoints on the circle. The diameter is twice the length of the radius.
• Circumference: The distance around the circle. It's calculated using the formula C = 2πr, where 'r' is the radius and π (pi) is a mathematical constant approximately equal to 3.14159.

Circles also possess other key components, such as chords (line segments with endpoints on the circle), tangents (lines that touch the circle at only one point), and secants (lines that intersect the circle at two points). However, for our purposes, the radius, diameter, and circumference are the most critical.

Understanding Arc Length

An arc is simply a portion of the circumference of a circle. Imagine taking a curved piece of the circle's edge; that's an arc. Arc length, then, is the distance along that curved portion.

Measuring Arc Length: Degrees and Radians

Arc length can be measured in two primary units: degrees and radians. A full circle encompasses 360 degrees. So, an arc that covers one-quarter of the circle would measure 90 degrees.

Radians, on the other hand, relate arc length directly to the circle's radius. One radian is defined as the angle subtended at the center of the circle by an arc whose length is equal to the radius of the circle.

A full circle measures 2π radians.

The Arc Length Formula

To calculate the arc length (s) of a circle, we use the following formula:

s = rθ

Where:

• s is the arc length
• r is the radius of the circle
• θ (theta) is the central angle (the angle formed at the center of the circle by the endpoints of the arc), measured in radians.

If the central angle is given in degrees, it must first be converted to radians before applying the formula. To convert from degrees to radians, multiply the angle in degrees by π/180.

Understanding arc length and its formula is crucial. It allows us to quantify portions of the circle's circumference. This quantification will prove essential when we delve into the concept of congruent arcs.

Central Angles and Intercepted Arcs: The Core Relationship

Having explored the fundamental properties of circles and arc lengths, we can now delve into a critical relationship that underpins the concept of congruent arcs: the connection between central angles and their intercepted arcs. This relationship is the key to unlocking a deeper understanding of arc measurement and congruence within circles.

Defining Central Angles and Intercepted Arcs

A central angle is an angle whose vertex lies at the center of a circle.

Its sides are formed by two radii of the circle.

An intercepted arc is the portion of the circle's circumference that lies within the central angle.

Imagine a slice of pizza; the angle formed at the center of the pizza is the central angle, and the crust of that slice represents the intercepted arc.

The Direct Proportionality

The relationship between a central angle and its intercepted arc is remarkably straightforward: the measure of the central angle (in degrees) is equal to the measure of its intercepted arc (in degrees).

This is a cornerstone principle in circle geometry.

For example, a central angle of 60° intercepts an arc that is also 60°.

Similarly, a 90° central angle intercepts a 90° arc, which represents one-quarter of the entire circle's circumference (360° / 4 = 90°).

Calculating Arc Measure

Understanding this direct proportionality is crucial for calculating arc measures.

If you know the measure of the central angle, you automatically know the measure of the intercepted arc, and vice versa.

This knowledge provides a powerful tool for solving geometric problems involving circles.

Illustrative Examples

Consider a circle with center O. If angle AOB is a central angle measuring 45°, then the intercepted arc AB also measures 45°.

Conversely, if we know that arc CD measures 120°, then the central angle COD that intercepts arc CD must also measure 120°.

This direct relationship simplifies calculations and allows for easy determination of unknown arc or angle measures, facilitating a deeper understanding of circle properties and laying the groundwork for exploring congruent arcs.

Having established the fundamental connection between central angles and intercepted arcs, it's time to explore the deeper theorems that solidify the relationship between congruent arcs and angles within a circle. These theorems provide the rigorous foundation needed to prove congruence and solve complex geometric problems. Understanding these principles unlocks the ability to deduce arc congruence based on angle relationships and vice versa.

Congruent Arcs and Angles: Theorems and Proofs

The geometry of circles hinges on precise relationships. Theorems are the tools we use to demonstrate and prove these relationships.

When dealing with congruent arcs, several key theorems connect them to angles, allowing us to deduce congruence based on angle measurements and positions within the circle.

Central Angles and Congruent Arcs

Central angles play a pivotal role in determining arc congruence.

The most fundamental theorem states: If two central angles in the same circle, or in congruent circles, are congruent, then their intercepted arcs are congruent.

This theorem is the cornerstone of many arc congruence proofs.

Imagine two identical circles with central angles of equal measure. The portions of the circumference "cut off" by these angles will necessarily be equal in length, hence congruent arcs.

Conversely, the theorem also holds in reverse: If two arcs in the same circle, or in congruent circles, are congruent, then their corresponding central angles are congruent.

This bidirectional relationship allows us to move seamlessly between angle measurements and arc lengths when assessing congruence.

Proving Congruence Using Central Angles

A typical proof using this theorem might proceed as follows:

1. Given: Circle O with central angles ∠AOB and ∠COD, where ∠AOB ≅ ∠COD.

2. Prove: Arc AB ≅ Arc CD.

3. Statement 1: ∠AOB ≅ ∠COD (Given).

4. Statement 2: m∠AOB = m∠COD (Definition of Congruent Angles).

5. Statement 3: mArc AB = m∠AOB; mArc CD = m∠COD (Measure of intercepted arc equals the measure of the central angle).

6. Statement 4: mArc AB = mArc CD (Substitution Property of Equality).

7. Statement 5: Arc AB ≅ Arc CD (Definition of Congruent Arcs).

This simple proof illustrates the direct application of the theorem and highlights the logical flow required for geometric proofs.

Inscribed Angles and Their Relationship to Congruent Arcs

Inscribed angles, whose vertices lie on the circle rather than at the center, also have a crucial relationship with intercepted arcs.

An inscribed angle intercepts an arc that is twice the measure of the angle.

This relationship opens another avenue for proving arc congruence.

The Inscribed Angle Theorem

The Inscribed Angle Theorem states: The measure of an inscribed angle is half the measure of its intercepted arc.

This means that if two inscribed angles intercept the same arc, they must be congruent.

Conversely, if two inscribed angles are congruent, they intercept congruent arcs.

Proving Congruence with Inscribed Angles

Suppose we have a circle with inscribed angles ∠AEB and ∠ADB both intercepting arc AB.

1. Given: Circle O with inscribed angles ∠AEB and ∠ADB intercepting arc AB.

2. Prove: ∠AEB ≅ ∠ADB.

3. Statement 1: ∠AEB intercepts arc AB; ∠ADB intercepts arc AB (Given).

4. Statement 2: m∠AEB = 1/2(mArc AB); m∠ADB = 1/2(mArc AB) (Inscribed Angle Theorem).

5. Statement 3: m∠AEB = m∠ADB (Transitive Property of Equality).

6. Statement 4: ∠AEB ≅ ∠ADB (Definition of Congruent Angles).

This proof elegantly demonstrates how the Inscribed Angle Theorem can be used to establish angle congruence based on a shared intercepted arc.

Furthermore, if we know that ∠AEB ≅ ∠ADB, we can conversely prove that the arcs they intercept within congruent circles are also congruent.

The theorems connecting central angles, inscribed angles, and intercepted arcs provide a powerful toolkit for analyzing and proving congruence within circles. By understanding these relationships, one can navigate complex geometric problems with greater confidence and precision.

Having explored the theorems that define the relationship between congruent arcs and angles, it's equally important to understand how we measure these arcs in the first place. Accurate measurement is essential for both proving congruence and applying these concepts in practical settings. This involves not only understanding the units of measurement but also the fundamental constant that governs circular relationships: Pi (π).

Radians, Degrees, and Pi: Measuring Arc Length Accurately

To work effectively with arcs, it's crucial to grasp the two primary units used to quantify them: degrees and radians. While degrees are a familiar concept from basic geometry, radians offer a more mathematically elegant way to express angles, particularly when dealing with arc length calculations. Understanding both, and how to convert between them, is key.

Degrees: A Familiar Unit

Degrees represent a fraction of a full circle, with 360 degrees comprising a complete rotation. This system, while convenient, is somewhat arbitrary. Its historical origins are linked to ancient Babylonian astronomy. Each degree can be further subdivided into 60 minutes, and each minute into 60 seconds, allowing for highly precise angular measurements.

Radians: Connecting Arc Length to Radius

Radians, on the other hand, are defined in terms of the circle's radius. One radian is the angle subtended at the center of a circle by an arc equal in length to the radius of the circle.

This definition directly links the angle to the physical dimensions of the circle.

This inherent relationship makes radians particularly useful in calculus and other advanced mathematical contexts involving circular functions.

Converting Between Radians and Degrees

The relationship between radians and degrees is based on the fact that a full circle (360 degrees) is equal to 2π radians. This gives us the following conversion formulas:

• To convert from degrees to radians: Multiply the angle in degrees by π/180.

• To convert from radians to degrees: Multiply the angle in radians by 180/π.

These conversions are essential for applying formulas and solving problems that involve both units of measurement. The ability to move seamlessly between these systems is a fundamental skill in geometry and trigonometry.

The Essential Role of Pi (π)

Pi (π) is the ratio of a circle's circumference to its diameter. It's an irrational number, meaning its decimal representation never ends and never repeats.

Its value is approximately 3.14159, but it's crucial to use the full precision available on your calculator or computer when performing calculations.

Pi is fundamental to all calculations involving circles, including arc length.

The circumference of a circle is given by the formula C = 2πr, where 'r' is the radius. Since arc length is a fraction of the circumference, Pi is integral to its calculation.

Arc Length Formula: Degrees vs. Radians

The arc length formula differs slightly depending on whether the angle is measured in degrees or radians.

• Degrees: Arc Length = (θ/360) * 2πr, where θ is the central angle in degrees and r is the radius.

• Radians: Arc Length = θr, where θ is the central angle in radians and r is the radius.

Notice how the radian formula is significantly simpler, highlighting the efficiency of using radians in mathematical calculations. By choosing the correct formula based on the given units, you can accurately determine the length of any arc within a circle.

Having explored the theorems that define the relationship between congruent arcs and angles, it's equally important to understand how these congruent arcs relate to other parts of the circle. This next part shifts our focus to chords, the line segments that connect the endpoints of an arc.

Congruent Arcs and Chords: Establishing Equivalence

The relationship between congruent arcs and chords represents another fundamental aspect of circle geometry. This relationship allows us to draw direct connections between arcs and the line segments that subtend them, opening up a new avenue for solving geometric problems. The core concept revolves around a powerful theorem: if two arcs in the same circle (or in congruent circles) are congruent, then their corresponding chords are also congruent, and vice versa.

The Congruent Arcs, Congruent Chords Theorem Explained

The theorem linking congruent arcs and congruent chords can be stated precisely as follows:

• If arc AB is congruent to arc CD, then chord AB is congruent to chord CD.
• Conversely, if chord AB is congruent to chord CD, then arc AB is congruent to arc CD.

This "if and only if" relationship signifies a bidirectional connection. Congruent arcs guarantee congruent chords, and congruent chords guarantee congruent arcs (within the same circle or congruent circles). The condition "within the same circle or congruent circles" is crucial, as the theorem doesn't necessarily hold true for arcs and chords in circles of different sizes.

Proving the Theorem

A formal proof of this theorem often involves demonstrating that triangles formed by the radii connecting the center of the circle to the endpoints of the arcs are congruent. If the central angles subtended by the arcs are congruent (due to the arcs being congruent), then the triangles formed are congruent by Side-Angle-Side (SAS) congruence. Consequently, the chords, being corresponding sides of congruent triangles, are also congruent. The converse can be proved similarly.

Practical Applications and Problem-Solving

The congruent arcs and chords theorem provides a valuable tool for problem-solving in geometry. It allows us to infer information about arcs from information about chords, and vice versa.

Let's consider a few examples:

• Finding Unknown Chord Lengths: If we know that two arcs in a circle are congruent and we know the length of the chord corresponding to one of the arcs, then we immediately know the length of the chord corresponding to the other arc.

• Determining Arc Congruence: If we are given that two chords in a circle are congruent, we can conclude that the arcs they subtend are also congruent. This can be useful in proving other geometric relationships or finding unknown angle measures.

• Symmetry Arguments: The theorem can be used in conjunction with symmetry arguments. For instance, if a diameter of a circle bisects a chord, then it also bisects the corresponding arc. This can be helpful in simplifying complex geometric figures.

• Geometric Constructions: Understanding the relationship between congruent arcs and chords is fundamental in geometric constructions. For example, copying an angle often relies on creating congruent arcs and chords.

Example Scenario:

Imagine a circular window divided into three equal sections by decorative bars. These bars are chords of the circle. Since the three sections are equal, the arcs between the bars are congruent.

Therefore, by the congruent arcs and chords theorem, the bars (chords) must also be of equal length. This principle could be used to verify the accurate and aesthetically pleasing design of the window.

Caveats and Considerations

While powerful, the theorem must be applied correctly. Remember these points:

• The theorem only applies to arcs and chords within the same circle or in congruent circles.
• Be mindful of major and minor arcs. If two chords are congruent, it is the minor arcs that are congruent.
• Always clearly state the theorem when using it in a proof.

By understanding and applying the congruent arcs and chords theorem, you gain another valuable tool in your geometric arsenal, enabling you to solve a wider range of problems and further appreciate the elegant relationships within circles.

Having established the fundamental theorems and relationships governing congruent arcs, it's crucial to explore their practical implications. Understanding congruent arcs isn't just an academic exercise; it's a gateway to solving real-world problems and understanding geometric proofs that underpin many aspects of our built environment.

Proofs and Real-World Applications of Congruent Arcs

The true power of geometric concepts lies in their ability to translate theoretical knowledge into tangible solutions. Congruent arcs are no exception. This section delves into examples of geometrical proofs where congruent arcs play a pivotal role. We'll also explore how this concept finds application in various real-world scenarios.

Geometrical Proofs Utilizing Congruent Arcs

Geometric proofs often rely on establishing congruence to deduce other properties. Congruent arcs, with their direct relationship to central angles and chords, provide a powerful tool for proving various geometric theorems.

For example, consider a scenario where two circles intersect at two points, A and B. If we can prove that the arcs intercepted by the line segment AB are congruent in both circles, then we can deduce various properties about the circles themselves, such as the equality of certain angles or the congruence of related triangles.

The key to successfully using congruent arcs in proofs is to carefully identify the relationships between arcs, angles, and chords. By applying the theorems discussed earlier, we can establish congruence and then use that congruence to reach further conclusions.

Real-World Applications of Congruent Arcs

The applications of congruent arcs extend far beyond the realm of pure mathematics. They are fundamental to various fields including engineering, architecture, and design.

Engineering

In engineering, congruent arcs are essential in the design of curved structures, such as bridges and arches. Ensuring that arcs are congruent guarantees uniformity and structural integrity.

For instance, the design of a suspension bridge involves careful calculations to ensure that the curved cables are perfectly shaped and uniformly loaded. Congruent arcs play a crucial role in achieving this uniformity.

Architecture

Architects use congruent arcs to create visually appealing and structurally sound designs. Arches, domes, and curved facades often incorporate congruent arcs to achieve symmetry and balance.

Consider the design of a rose window in a cathedral. The intricate patterns often involve congruent arcs and circles, creating a harmonious and aesthetically pleasing design.

Design

In design, congruent arcs are used to create symmetrical and balanced patterns. This is especially important in fields such as graphic design and industrial design.

From designing logos to creating aesthetically pleasing product shapes, congruent arcs ensure visual harmony and professional appeal. They contribute to the overall perception of balance and intentionality in design work.

Analyzing Congruent Arcs Using Euclidean Geometry Principles

Euclidean geometry provides the foundational principles for understanding and analyzing congruent arcs. Key concepts include:

• Postulates of Congruence: These postulates define the conditions under which two geometric figures are considered congruent.
• Angle Relationships: Understanding the relationships between central angles, inscribed angles, and intercepted arcs is crucial for proving congruence.
• Circle Properties: Properties such as the equality of radii in a circle are essential for establishing congruence between arcs.

By applying these Euclidean principles, we can rigorously analyze congruent arcs and use them to solve geometric problems. Understanding these foundational principles will empower you to use congruent arcs effectively in a variety of contexts. Mastery of these concepts opens a world of possibilities in both theoretical and practical applications of geometry.

Having shown how congruent arcs manifest in geometric proofs and practical applications, it's beneficial to briefly touch upon their relationship with other advanced circle components. These connections provide a broader understanding of circular geometry and highlight the interwoven nature of these concepts.

Advanced Concepts: Secants, Tangents, and Beyond

While a deep dive into secants and tangents is beyond the scope of this core exploration of congruent arcs, understanding their basic relationships enriches our perspective. These lines, interacting with circles in specific ways, also form angles and arcs that can be related to congruent arcs.

Secants and Intercepted Arcs

A secant is a line that intersects a circle at two distinct points. When two secants intersect inside or outside the circle, they intercept arcs on the circle.

The angles formed by these intersecting secants are related to the measures of the intercepted arcs. The relationships are defined by specific theorems.

For example, if two secants intersect inside a circle, the measure of the angle formed is equal to one-half the sum of the measures of the intercepted arcs.

If the secants intersect outside the circle, the angle's measure is equal to one-half the difference of the intercepted arcs.

Understanding these relationships can help us determine when arcs are congruent, based on the angles formed by secants.

Tangents and Points of Tangency

A tangent is a line that touches a circle at exactly one point, called the point of tangency. The radius of the circle drawn to the point of tangency is always perpendicular to the tangent line.

When a tangent and a secant intersect at the point of tangency, the angle formed is equal to one-half the measure of the intercepted arc.

If two tangents intersect outside the circle, the angle formed by the tangents is related to the intercepted major and minor arcs.

Specifically, the angle is half the difference between the major and minor arc measures.

These relationships can indirectly assist in identifying congruent arcs. Determining angle measures using tangents allows us to deduce the measures of related arcs.

Beyond the Basics: Connecting Concepts

The principles governing secants and tangents are rooted in Euclidean geometry and build upon fundamental concepts like central angles and intercepted arcs.

While not directly proving arc congruence themselves, understanding the relationships between secants, tangents, and intercepted arcs gives a more complete picture of the geometry of circles.

Exploring these concepts can provide a deeper appreciation for how geometric elements interact within and around a circle.

These connections ultimately show the broader application of geometric principles beyond isolated theorems about congruent arcs.

Video: Master Congruent Arcs: Geometry's Secret Weapon!

Play Video