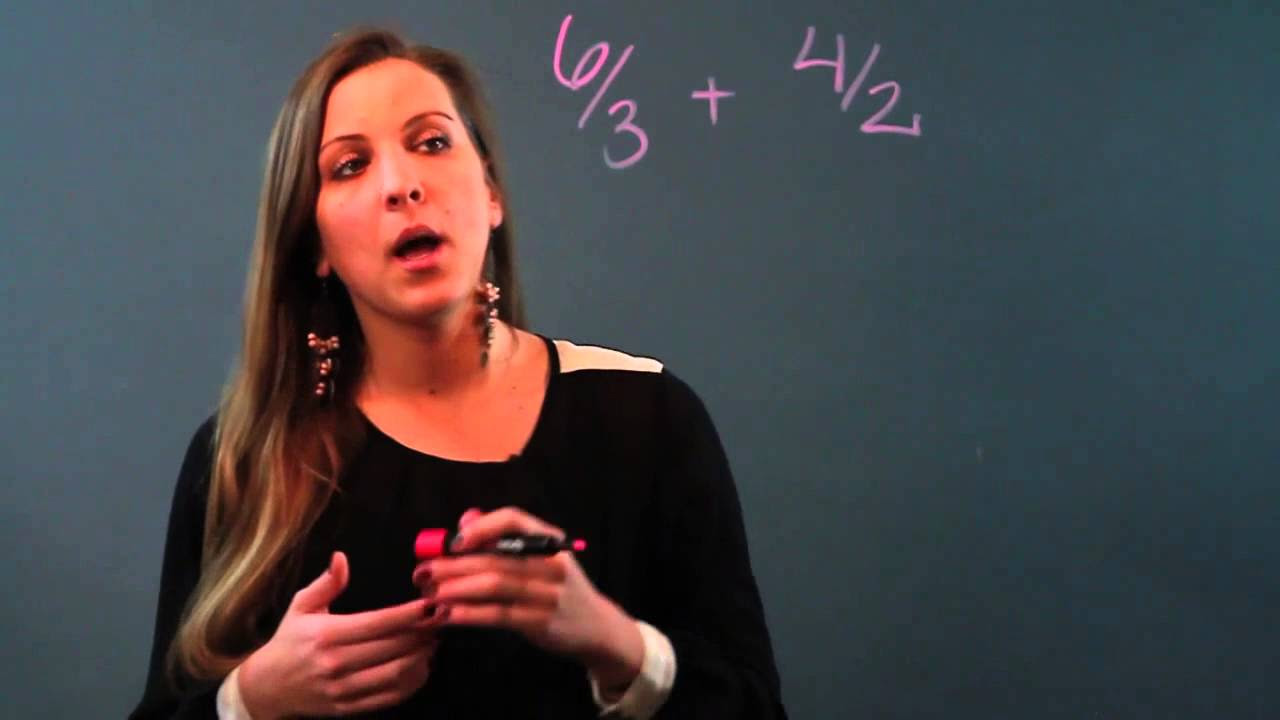Fractions as Integers: The Shocking Truth Will Surprise You!

Understanding number theory requires discerning the relationships between different numerical sets. Rational numbers, a fundamental concept, encompass ratios expressed as fractions, bringing us to the critical question: are fractions integers? Euclidean geometry, with its foundational axioms, establishes the basis for understanding numerical properties. Examining the principles taught within Khan Academy regarding number systems further clarifies the distinctions and overlaps. Consequently, investigating these interconnected entities is crucial to grasp whether fractions truly fall under the umbrella of integers, or not.

Image taken from the YouTube channel ehow , from the video titled How to Balance Equations With Positive and Negative Integers & Fractions : Fractions 101 .

For many, fractions and integers exist in separate compartments of the mathematical mind. Integers are seen as whole, complete entities, while fractions represent broken or partial quantities. This initial perception, while understandable, obscures a deeper and more nuanced relationship.

While it’s true that the vast majority of fractions do not neatly translate into integers, the core idea of this piece is that some fractions undeniably are integers. The exploration of fractions, integers, and the broader category of rational numbers reveals that the lines are more blurred than many realize. We aim to demonstrate how these seemingly distinct concepts are intimately connected.

Challenging the Divide

The common misconception stems from how we're initially introduced to these numbers. Integers are often presented as the building blocks of counting – 1, 2, 3, and so on. Fractions, on the other hand, arrive later, often accompanied by the notion of dividing a pizza or sharing a cake.

This creates a mental separation, a sense that fractions are somehow less than integers, or that they belong to an entirely different numerical realm. It is this perceived separation that we will challenge and, ultimately, bridge.

The Thesis: Fractions Can Be Integers

The central argument is straightforward: Although most fractions do not represent integers, there exists a subset of fractions that, when simplified, yield whole numbers.

This isn’t merely a semantic trick. It reflects a fundamental property of rational numbers and the division operation they represent.

A Roadmap for Understanding

To fully grasp this relationship, we will navigate a clear and structured path:

First, we will establish precise definitions of integers, fractions, and rational numbers. This will ensure that everyone is operating from the same foundation of understanding.

Second, we will delve into concrete examples. We will examine fractions that simplify to integers and fractions that do not, illustrating the key differences between the two.

Finally, we will explore the conditions under which a fraction becomes an integer. By outlining these conditions, we can develop a predictive understanding of when this transformation occurs.

Challenging the common misconception that integers and fractions exist as distinct mathematical entities requires a solid foundation in their definitions. Understanding these concepts is crucial for appreciating the subtle connections between them. Therefore, before we delve deeper into fractions that are integers, let’s establish clear definitions for the key terms at play: integers, fractions, and rational numbers.

Defining the Building Blocks: Integers, Fractions, and Rational Numbers

To truly understand how fractions can sometimes be integers, we must first define our terms with precision. These definitions provide the framework for exploring the relationship between these numerical concepts.

What is an Integer?

Integers form the bedrock of our number system. An integer is defined as any whole number, whether it's positive, negative, or zero. There are no fractional or decimal components.

Examples include: -3, -2, -1, 0, 1, 2, 3, and so on.

Integers can be visually represented on a number line. Zero sits at the center, with positive integers extending infinitely to the right and negative integers extending infinitely to the left.

Each integer occupies a distinct point on the line, with a clear and defined distance between each number.

What is a Fraction?

A fraction represents a part of a whole. It's expressed as a ratio between two numbers: a numerator and a denominator.

The numerator indicates the number of parts we have, while the denominator indicates the total number of equal parts that make up the whole.

For example, in the fraction 1/2, "1" is the numerator, and "2" is the denominator. This fraction represents one part out of a total of two equal parts. Other examples include 3/4 (three parts out of four) and 5/7 (five parts out of seven).

What are Rational Numbers?

Rational numbers encompass a broader category. A rational number is any number that can be expressed as a fraction p/q, where both p and q are integers, and q is not equal to zero. The condition that q cannot be zero is vital; division by zero is undefined in mathematics.

Importantly, integers themselves can be expressed as fractions. Any integer 'n' can be written as n/1. For instance, the integer 5 can be expressed as the fraction 5/1. This demonstrates that integers are a subset of rational numbers. Understanding this inclusion is key to seeing how certain fractions can resolve into integers.

To truly appreciate how integers and fractions intertwine, we established clear definitions in the previous section. Understanding what constitutes an integer, a fraction, and a rational number lays the groundwork for a critical realization: a fraction is, at its core, a representation of division. Let's explore how this seemingly simple concept bridges the gap between these two numerical realms.

Division: The Bridge Connecting Fractions and Integers

The fraction bar, often seen as merely a notational convention, carries a profound meaning. It's a symbol that explicitly instructs us to perform a division operation.

The numerator is the dividend, the number being divided, while the denominator is the divisor, the number doing the dividing. Recognizing this fundamental connection unlocks a deeper understanding of when a fraction transforms into an integer.

Fractions as Division

Think of the fraction 3/4. This isn't just some abstract concept. It's a clear instruction: divide 3 by 4. The result, 0.75, is not an integer, but the act of division is undeniably present.

Similarly, 1/2 instructs us to divide 1 by 2, yielding 0.5, again a non-integer result.

The key takeaway is that every fraction inherently embodies a division problem, whether the result is an integer or not.

When Does Division Result in an Integer?

The crucial question then becomes: under what conditions does this division yield a whole number, or an integer? The answer lies in the concept of divisibility.

The Role of Divisibility

If the numerator is perfectly divisible by the denominator, meaning the division results in a whole number with no remainder, then the fraction simplifies to an integer.

Consider the fraction 6/3. When we perform the division, 6 divided by 3 equals 2. There is no remainder. Therefore, 6/3 is equivalent to the integer 2.

Another example is 10/2. 10 divided by 2 equals 5, again with no remainder. Consequently, 10/2 simplifies to the integer 5.

In essence, a fraction is an integer when the numerator is a multiple of the denominator.

Visualizing Fractions as Integers on the Number Line

The number line offers a powerful visual aid for understanding this concept. If we plot fractions like 4/2 or 6/3 on the number line, we'll observe something significant.

4/2, which simplifies to 2, lands precisely on the point representing the integer 2. Similarly, 6/3, which simplifies to 2, also lands on the integer 2.

This illustrates that fractions that are integers don't occupy spaces "between" integers on the number line. Instead, they coincide perfectly with existing integer points.

The number line provides visual confirmation that certain fractions are, in fact, integers in disguise. They are simply expressed in fractional form before being simplified to their whole number equivalent.

To truly understand the dance between fractions and integers, it’s helpful to move beyond abstract definitions and delve into concrete examples. Examining specific cases where fractions either do or do not simplify to integers solidifies the concepts we've explored. This section provides a curated list of fractions, dissected to reveal their inherent relationship with the world of integers.

Examples: Fractions That Are and Are Not Integers

The beauty of mathematics lies in its ability to be demonstrated through tangible examples. By looking at fractions that resolve into integers and those that do not, we can solidify our understanding of the principles of divisibility and the fundamental connection between these numerical forms.

Fractions That Are Integers: A Matter of Divisibility

These fractions, at first glance, appear to be standard fractions. However, they possess a crucial property: their numerators are multiples of their denominators. This perfect divisibility is the key to their integer transformation.

Consider the fraction 8/2. This represents 8 divided by 2, which equals 4. Since 4 is a whole number and an integer, 8/2 qualifies as a fraction that is also an integer.

Similarly, 12/4 simplifies to 3, another integer. And 15/5 resolves to 3 as well.

More examples of fractions that are integers include:

• 20/5 = 4
• 21/3 = 7
• 36/6 = 6
• 100/10 = 10

The common thread? Each numerator is cleanly divisible by its corresponding denominator, leaving no remainder and resulting in a whole number.

It's the seamless division that allows these fractions to cross over into the realm of integers.

Fractions That Are Not Integers: The Remainder's Tale

Now, let's shift our focus to fractions that stubbornly refuse to become integers. These are the fractions where the numerator is not perfectly divisible by the denominator.

Take the quintessential example, 1/2. Dividing 1 by 2 yields 0.5. This is a decimal, a number that exists between integers, and therefore not an integer itself.

Likewise, 3/4 results in 0.75. 5/7 produces approximately 0.714.

Here are some additional examples of fractions that aren't integers:

• 2/3 = 0.666...
• 4/5 = 0.8
• 7/8 = 0.875
• 11/2 = 5.5

In all these cases, the division operation leaves a remainder, pushing the result outside the boundaries of the integer world. These fractions represent quantities that fall between whole numbers, maintaining their fractional identity.

Flipping the Script: Representing Whole Numbers as Fractions

Having explored how certain fractions neatly resolve into integers, it's time to reverse our perspective. This section examines how integers themselves can be expressed as fractions, solidifying the understanding that these seemingly distinct numerical concepts are, in fact, deeply intertwined.

The Integer as a Fraction: n/1

Any integer, let's call it n, can be written as a fraction in the form of n/1.

This might seem like a trivial observation, but it carries significant weight.

Consider the number 5. We can express it as 5/1 without altering its value. The same applies to -3, which can be written as -3/1, or even 0, expressed as 0/1.

The denominator of 1 acts as a silent operator, preserving the integer's inherent value.

Whole Numbers: A Subset of Rational Numbers

Expressing integers as fractions (with a denominator of 1) highlights a crucial point: whole numbers and integers are, in fact, subsets of rational numbers.

Remember, rational numbers are defined as any number that can be expressed in the form p/q, where p and q are integers, and q is not zero.

Since we can represent any integer n as n/1, it inherently fulfills the criteria of a rational number.

This perspective further emphasizes that integers are a special case within the broader realm of rational numbers.

Implications and Significance

Understanding that integers can be represented as fractions clarifies their place within the number system. It reinforces the notion that mathematical concepts are interconnected, not isolated entities.

This understanding becomes particularly valuable when performing algebraic manipulations, simplifying expressions, or working with complex equations where recognizing equivalent forms is key to problem-solving.

Having established the fundamental connection between integers and fractions, it's natural to question the practical value of this understanding. Why dedicate time to exploring what might seem like a purely theoretical concept? The answer lies in the profound impact this knowledge has on our mathematical fluency and problem-solving abilities.

The Significance: Why Understanding This Matters

The realization that fractions can be integers, and integers can be fractions, isn't just a quirky mathematical fact; it's a key that unlocks a deeper comprehension of the number system itself. This understanding has significant implications for mathematical proficiency, particularly in advanced topics like algebra and calculus.

Building a Robust Foundation in Number Sense

Understanding the relationship between integers and fractions fosters a more flexible and intuitive number sense.

It allows us to move beyond rote memorization of rules and procedures, enabling us to reason more effectively about numerical relationships.

By recognizing that seemingly disparate concepts are, in fact, interconnected, we develop a more holistic understanding of mathematics.

This robust foundation is crucial for tackling more complex mathematical challenges.

The Power of Rational Numbers

Central to grasping the relationship between integers and fractions is a thorough understanding of rational numbers.

Rational numbers encompass all numbers that can be expressed as a fraction, p/q, where p and q are integers and q is not zero.

This definition reveals that integers are simply a specific type of rational number where the denominator is 1.

Comprehending this hierarchical relationship allows for seamless transitions between different number representations.

Ultimately building confidence in manipulating and comparing numbers in various forms.

Facilitating Algebraic and Calculus Manipulations

The ability to fluidly move between integer and fractional representations proves invaluable when navigating the complexities of algebra and calculus.

For example, simplifying algebraic expressions often requires converting integers into fractions with a common denominator.

Similarly, integrating certain functions in calculus may involve rewriting integers as fractions to facilitate the application of specific integration techniques.

Furthermore, understanding the underlying principles behind these manipulations prevents reliance on memorization.

It encourages a more conceptual approach to problem-solving.

Real-World Applications

The abstract concepts discussed translate into tangible benefits in various real-world scenarios.

From calculating proportions in cooking to determining financial ratios in business, the ability to seamlessly work with fractions and integers is essential.

Understanding these relationships enhances problem-solving skills across diverse fields.

It allows us to approach quantitative challenges with greater confidence and accuracy.

Video: Fractions as Integers: The Shocking Truth Will Surprise You!

Play Video

So, after all that deep diving, hopefully things are a little clearer when you ponder whether are fractions integers. Keep those numbers crunching and stay curious!