Secrets of Compressional Waves: Explained Simply!

A compressional wave, also known as a longitudinal wave, is a fascinating phenomenon explored extensively in acoustics, the science of sound. These waves, unlike transverse waves, cause particles in a medium to oscillate parallel to the direction of energy propagation. Understanding the behavior of a compressional wave is crucial for various applications, from medical imaging using ultrasound technology to geophysical explorations conducted by organizations such as the United States Geological Survey (USGS). Even the design of musical instruments, where the manipulation of sound waves is paramount, depends on the precise characteristics of this type of wave.

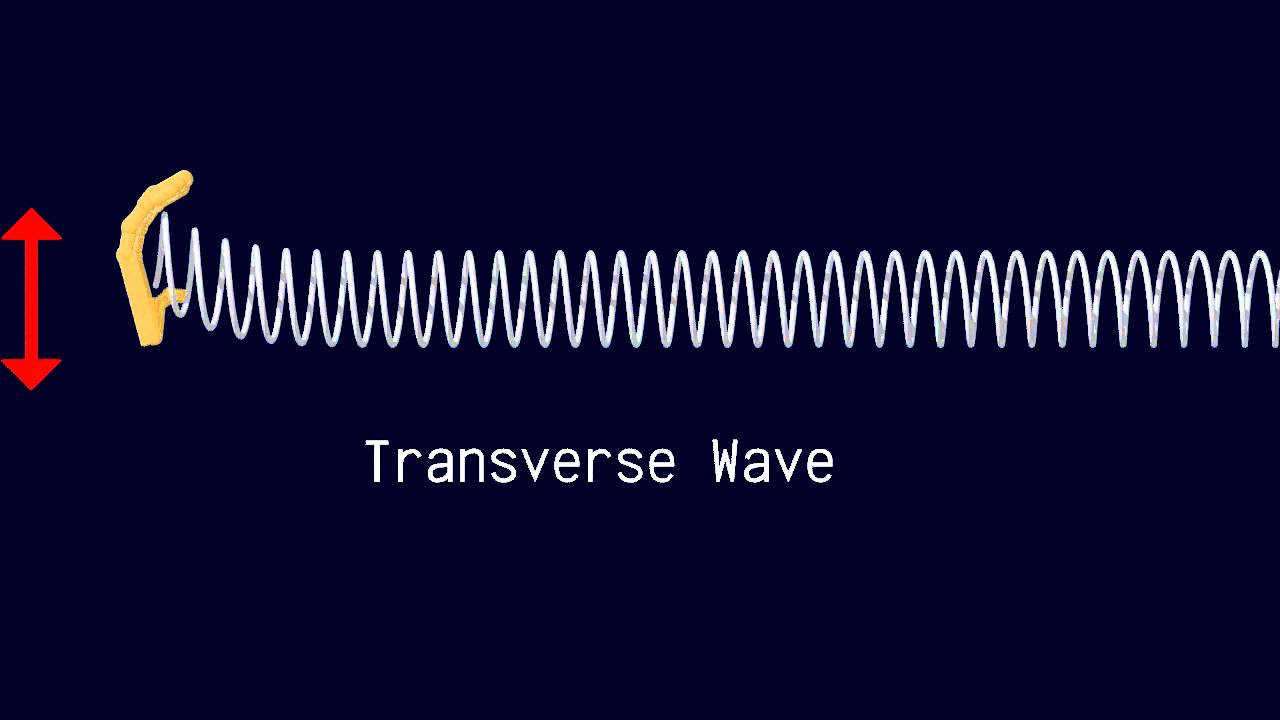
Image taken from the YouTube channel Animations for Physics and Astronomy , from the video titled Longitudinal and Transverse Waves .

Imagine the earth trembling beneath your feet, a distant rumble growing into a deafening roar. Or perhaps you are enjoying the rich, vibrant notes of a symphony. What connects these seemingly disparate experiences? The answer lies in compressional waves, unseen yet profoundly influential forces shaping our perception of the world around us. These waves, also known as longitudinal waves, are responsible for transmitting energy through various media, creating the phenomena we experience as sound, seismic activity, and much more.

Let's begin with the captivating world of sound. Every note you hear, every word spoken, travels to your ears as a compressional wave. These waves are created by vibrating objects, like a guitar string or a human vocal cord, which disturb the surrounding air molecules. These disturbances then propagate outwards as areas of compression and rarefaction, ultimately reaching your eardrum and allowing you to perceive sound.

Alternatively, consider the immense power unleashed during an earthquake. When tectonic plates shift and collide deep within the Earth, they generate seismic waves that radiate outwards in all directions. Among these seismic waves are P-waves, or primary waves, which are compressional in nature. These waves travel faster than other types of seismic waves and can traverse through solids, liquids, and gases, providing valuable information about the Earth's internal structure.

These examples demonstrate the ubiquitous presence and importance of compressional waves in our daily lives and in the grand scheme of geological processes.

Thesis Statement: Exploring the Nature and Significance

This article will delve into the fundamental principles of compressional waves, exploring their nature as mechanical waves and examining how they propagate through different media. We will also investigate the critical role compressional waves play in various scientific and technological applications, from understanding the intricacies of human hearing to probing the depths of our planet. Finally, we will discuss some of the real-world applications. By the end of this exploration, you will gain a deeper appreciation for the hidden forces that shape our world through the fascinating realm of compressional waves.

Imagine the earth trembling beneath your feet, a distant rumble growing into a deafening roar. Or perhaps you are enjoying the rich, vibrant notes of a symphony. What connects these seemingly disparate experiences? The answer lies in compressional waves, unseen yet profoundly influential forces shaping our perception of the world around us. These waves, also known as longitudinal waves, are responsible for transmitting energy through various media, creating the phenomena we experience as sound, seismic activity, and much more.

Let's begin with the captivating world of sound. Every note you hear, every word spoken, travels to your ears as a compressional wave. These waves are created by vibrating objects, like a guitar string or a human vocal cord, which disturb the surrounding air molecules. These disturbances then propagate outwards as areas of compression and rarefaction, ultimately reaching your eardrum and allowing you to perceive sound.

Alternatively, consider the immense power unleashed during an earthquake. When tectonic plates shift and collide deep within the Earth, they generate seismic waves that radiate outwards in all directions. Among these seismic waves are P-waves, or primary waves, which are compressional in nature. These waves travel faster than other types of seismic waves and can traverse through solids, liquids, and gases, providing valuable information about the Earth's internal structure.

These examples demonstrate the ubiquitous presence and importance of compressional waves in our daily lives and in the grand scheme of geological processes. Now, to truly grasp the nature of these phenomena, we must delve into the fundamental physics that govern their behavior.

Understanding the Basics: Compressional Waves as Longitudinal Waves

At their core, compressional waves represent a specific type of mechanical wave where the particle displacement is parallel to the direction of wave propagation. This characteristic places them squarely within the broader category of longitudinal waves. To fully understand their behavior, it's essential to dissect their defining features and how they interact with the medium through which they travel.

Compressional Waves: A Longitudinal Perspective

The terms "compressional wave" and "longitudinal wave" are often used interchangeably, and for good reason. The defining characteristic of both is that the oscillation of particles in the medium occurs in the same direction as the wave's movement.

Consider a Slinky. If you push and pull one end, you'll create areas where the coils are bunched together (compressed) and areas where they are spread apart (rarefied). This push-and-pull motion transmits the energy along the Slinky's length, demonstrating a longitudinal wave in action.

Similarly, compressional waves in air, water, or solids cause the molecules to vibrate back and forth along the path of the wave. The key takeaway here is the direction of particle motion. Unlike transverse waves, where the particles move perpendicular to the wave's direction (like a wave on a string), compressional waves rely on parallel motion.

The Dance of Compressions and Rarefactions

The propagation of a compressional wave is characterized by the alternating formation of compressions and rarefactions.

Compressions are regions where the particles of the medium are forced closer together, resulting in an area of increased density and pressure.

Rarefactions, conversely, are regions where the particles are spread further apart, leading to decreased density and pressure.

Imagine a piston rapidly pushing and pulling on air inside a cylinder. Each push creates a compression, forcing air molecules closer together. Each pull creates a rarefaction, allowing the air molecules to spread out. These alternating zones of high and low pressure then propagate down the cylinder, carrying the energy of the piston's movement. This rhythmic dance of compressions and rarefactions is what enables compressional waves to transmit energy through a medium.

Key Characteristics: Defining Wave Properties

To fully describe a compressional wave, we need to consider its key characteristics: wavelength, amplitude, and frequency. These properties determine the wave's behavior and how we perceive it (especially in the context of sound).

Wavelength

Wavelength (λ) is defined as the distance between two successive compressions or two successive rarefactions. It's a measure of the spatial extent of one complete wave cycle.

A shorter wavelength corresponds to a higher-pitched sound, while a longer wavelength corresponds to a lower-pitched sound.

Amplitude

Amplitude is related to the maximum displacement of particles from their resting position. In the context of sound, amplitude corresponds to the intensity or loudness of the sound. A higher amplitude wave carries more energy and will be perceived as louder.

Frequency

Frequency (f) refers to the number of complete wave cycles that pass a given point per unit of time, typically measured in Hertz (Hz). One Hertz equals one cycle per second. In sound, frequency determines the pitch of the sound. A higher frequency corresponds to a higher pitch, while a lower frequency corresponds to a lower pitch.

Wave Velocity: Factors Influencing Speed

The velocity (v) of a compressional wave, or how fast it travels through a medium, is influenced by several factors, most notably the properties of the medium itself. The relationship between wave speed, frequency, and wavelength is defined by the equation: v = fλ.

The speed of a compressional wave is directly related to the medium's elasticity and inversely related to its density. A more elastic medium allows the wave to propagate faster, while a denser medium slows it down. This is why sound travels much faster in solids than in liquids or gases, as solids are generally more elastic and have higher densities. Similarly, the speed of sound in air increases with temperature because the increased kinetic energy of the air molecules allows them to transmit the compressions and rarefactions more quickly.

To truly grasp the nature of these phenomena, we must delve into the critical role that the medium plays in the transmission of compressional waves. Without a medium, be it solid, liquid, or gas, these waves simply cannot propagate, highlighting the intimate relationship between the wave and its environment.

The Role of the Medium: Transmission Through Solids, Liquids, and Gases

Compressional waves, unlike electromagnetic waves such as light or radio waves, are mechanical waves. This distinction is fundamental to understanding their behavior.

Mechanical waves require a medium through which to travel because their energy is transferred via the interaction of particles within that medium. This means that compressional waves cannot exist in a vacuum.

The Necessity of a Medium

The existence of a medium is not just helpful, it is absolutely essential for the propagation of compressional waves. The wave's energy is passed from one particle to the next through collisions and interactions.

Without particles to interact with, there is no mechanism for the wave to move forward. Imagine trying to start a wave in an empty stadium; it’s the crowd (the medium) that allows the wave to travel.

Wave Speed and the Properties of the Medium

The velocity of a compressional wave is highly dependent on the properties of the medium through which it travels. Key factors include the medium's density and elasticity.

Density refers to the mass per unit volume of the medium. Elasticity describes the medium's ability to return to its original shape after being deformed.

Generally, compressional waves travel faster through denser and more elastic materials.

For instance, sound travels much faster through steel than through air, because steel is both denser and more elastic.

Speed Variations Across Different States of Matter

The state of matter (solid, liquid, or gas) profoundly influences wave speed.

Compressional waves generally travel fastest in solids, followed by liquids, and then gases.

This is primarily due to the differences in the arrangement and interaction of molecules in each state.

In solids, molecules are tightly packed and strongly bonded, facilitating efficient energy transfer.

Liquids have molecules that are less tightly packed and have weaker bonds than solids, resulting in slower wave speeds.

Gases have the least dense molecular arrangement with minimal interactions, leading to the slowest propagation speeds.

For example, the speed of sound in air is approximately 343 m/s, while in water it is around 1480 m/s, and in steel, it can reach speeds of about 5960 m/s.

Wave Propagation in Different Medium Types

Solids

In solids, the close proximity of atoms allows for efficient transmission of compressional waves. The elasticity of the solid plays a significant role; a stiffer material will generally transmit waves faster.

However, imperfections and grain boundaries within a solid can scatter the wave, potentially reducing its energy.

Liquids

Compressional waves in liquids are affected by the liquid's density and bulk modulus (a measure of its resistance to compression).

Liquids can support compressional waves because their molecules can be compressed and expanded, but they cannot support shear waves (transverse waves).

Temperature also affects the speed of sound in liquids; warmer liquids generally have lower densities and slower sound speeds.

Gases

In gases, the speed of compressional waves is primarily determined by the gas's temperature and molecular mass.

Higher temperatures increase the kinetic energy of the gas molecules, leading to faster wave propagation.

Lighter gases tend to have higher sound speeds than heavier gases at the same temperature.

Generally, the denser a medium, the slower the wave travels. Conversely, the more elastic a medium, the faster the wave travels. These relationships create a diverse range of compressional wave speeds across different materials, impacting how these waves behave in various environments. But the theoretical knowledge only takes us so far.

Compressional Waves in Action: Real-World Applications Explored

The study of compressional waves transcends the realm of theoretical physics, manifesting in a myriad of real-world applications that shape our understanding of the world around us and drive technological advancements.

From the simple act of hearing to the complex science of earthquake prediction and medical diagnostics, compressional waves play a pivotal role.

Sound and Hearing: The Symphony of Compressional Waves

Perhaps the most familiar application of compressional waves lies in the realm of sound. Sound, at its core, is a compressional wave.

When an object vibrates, it creates areas of high pressure (compressions) and low pressure (rarefactions) in the surrounding air.

These pressure variations propagate outwards as a compressional wave.

Our ears are exquisitely sensitive to these pressure fluctuations.

The eardrum vibrates in response to the incoming compressional wave, setting off a chain reaction that ultimately translates into the perception of sound in our brains.

The frequency of the wave determines the pitch of the sound, while the amplitude corresponds to its loudness.

The intricate mechanisms of our auditory system allow us to decode the complex information carried by these waves, enabling us to communicate, appreciate music, and navigate our environment.

Seismic Waves and Earthquakes: Unveiling Earth's Secrets

Compressional waves also play a critical role in seismology, the study of earthquakes and the Earth's interior.

Earthquakes generate various types of seismic waves, including P-waves (Primary waves), which are compressional waves.

P-waves are characterized by their ability to travel through solids, liquids, and gases, making them invaluable tools for probing the Earth's structure.

Seismologists use the arrival times and characteristics of P-waves to determine the location and magnitude of earthquakes.

Furthermore, by analyzing how P-waves refract and reflect as they pass through different layers of the Earth, scientists can deduce the composition and properties of these layers.

This includes the mantle and core.

The speed of P-waves is affected by the density and elasticity of the materials they traverse, providing insights into the Earth's internal structure.

For example, a sudden change in wave speed can indicate a boundary between different layers.

Beyond Sound and Seismology: A Spectrum of Applications

The utility of compressional waves extends far beyond the realms of sound and seismology.

Medical imaging relies heavily on ultrasound, which utilizes high-frequency compressional waves to create images of internal organs and tissues.

Unlike X-rays, ultrasound is non-ionizing and considered safe for repeated use.

In sonar (Sound Navigation and Ranging), compressional waves are emitted underwater to detect objects and map the seabed.

The time it takes for the waves to return after reflecting off an object is used to calculate its distance and size.

Industrially, compressional waves are employed in non-destructive testing to detect flaws and imperfections in materials without causing damage.

These techniques are crucial in ensuring the safety and reliability of structures and components in various industries, from aerospace to manufacturing.

These diverse applications underscore the versatility and importance of compressional waves in modern science and technology.

Video: Secrets of Compressional Waves: Explained Simply!

Play Video

So, there you have it – a peek behind the curtain of compressional waves! Hopefully, you now have a better understanding of how these waves work. Go forth and explore the world of sound and vibration; you might be surprised by what you discover!